Both radar and communication systems are RF systems that can be combined with a partially shared technology basis. The challenge is to combine them with the aim of improving radar performance. A network of radar stations, where each station would operate either mono-statically or bi-statically, could be used to ensure that targets are viewed from different aspect angles, allowing classification of objects. The abundance of different information about the targets has to be communicated by the individual radar stations. The information exchange is possible by using a centralized or distributed solution by wireless transmission from one station to the other. The communications among multiple radar units can be embedded in the radar signal without extra infrastructure, allowing in this way the exchange of communication messages between radar stations, including the reports of detected targets, for example.
However, there are fundamental differences between communications and radar systems. Communication needs higher signal-to-noise ratios (SNR) at the receiver for proper recognition of the transmitted symbols, while radar systems can integrate over a specified number of transmitted pulses. Time-varying multipath channels pose problems to the communication systems due to moving objects, while for radar this is a basic feature for recognition of moving targets.1 In this way, the proper selection of a specific waveform can alleviate those issues and facilitate the combination between communications and radar.
According to the waveform, both systems typically desire a large time-bandwidth product and an efficient use of the spectral resources. Nevertheless, radar systems also have other challenges due to their frequent operation in more complex environments; for example, unmasking weaker targets in a multi-target scenario or solving range/Doppler ambiguities.
This article is focused on a mono-static single radar station belonging to a certain network, where the communication signal is embedded in the radar transmit waveform, in this case OFDM. Range and Doppler processing schemes proposed by some of the authors will be tested to evaluate the radar operation with such a signal. Moreover, the feasibility and demonstration that OFDM waveforms can provide both communications among radar stations and radar operation, even when high computational complexity appears in the processing schemes, will be shown and verified with the results obtained from an extensive set of measurements. Synchronization among stations, network structure and physical-layer assessment is beyond the scope of this report; information on these topics can be found in the literature.2
Waveforms Generated
The concepts described in the following are developed in the context of a study of the viability and the opportunities rising from a wireless network of radars supporting an integrated communication link on the radar transmit signal. The objective is to prove the double usage (radar and communications) of specifically designed radar waveforms, such as OFDM, taking the limitations of current electronic devices and equipment capabilities into account.
The waveform selected for the synergy between radar and communication is OFDM. Recently, there has been a lot of interest in OFDM signals, not only for communication but also for radar. For this, OFDM has been studied extensively.3-6 Wideband radar systems are easily obtained with OFDM, where the spacing between carriers can be chosen to be large enough, obtaining in this way a large instantaneous bandwidth to provide the radar with a higher resolution capability (ability to distinguish between two or more targets on the same bearing at different ranges).
OFDM is a digital multi-carrier transmission technique that allows an efficient use of the bandwidth and a simultaneous large instantaneous bandwidth for the radar operation. This modulation scheme maps the digitally encoded symbols over several frequencies (subcarriers) to achieve robustness against fading in a multipath radio channel. Even though the spectra of the individual subcarriers overlap, the information can be completely recovered without any interference from other subcarriers as a consequence of the orthogonality of the base functions of the Fourier series.6 In a multipath scenario, orthogonality is kept among subcarriers by inserting a cyclic prefix (usually a cyclic extension of the current transmit symbol). However, the addition of this prefix, which mitigates the effects of link fading and inter-symbol interference (ISI), increases the bandwidth and introduces some loss in efficiency since no new information is carried. Moreover, for communications purposes, OFDM permits frequency diversity improving the reliability of a message signal by using two or more communications channels with different characteristics. In this way it is possible to combat co-channel interference and avoid error bursts.7
Nevertheless, several challenges arise for the novel use of this multi-carrier waveform in radar. The range and Doppler processing are different compared to standard processing schemes in order to benefit from the characteristics that the OFDM waveform offers, such as tunability and individual subcarrier retrieval. The flexibility in the coding of the OFDM waveform for communication purposes requires an ad hoc processing scheme to counteract interference between close-by object targets in the radar processing. The large instantaneous bandwidth required for radar operation imposes challenges to standard electronic equipment. There are more stringent requirements on the waveform for radar operation than for communication. Signal parameters such as subcarriers spacing Δƒ, and number of possible subcarriers N inserted in a specified bandwidth, require careful consideration to preserve orthogonality among subcarriers and prevent a high peak to average power ratio (PAPR), since the latter is proportional to the number of subcarriers if their phases were perfectly aligned.
The communication encoding of the OFDM waveform has to be properly designed in order to constrain the inherent high PAPR of this signal. This measure allows the linear operation of the amplifiers in the receive chain to prevent signal distortion and the optimal use of the dynamic range of the global system. The PAPR of the OFDM waveforms was limited by constraining the random phase codes, simulating the communication messages. Golay codes are also a valid alternative to constrain the PAPR in OFDM signals. Nevertheless, a trade off between communication throughput and radar performance comes up.8 In this way, the PAPR of the OFDM waveforms used along the experiments can be limited to values smaller than 10 dB or even until 3 dB.
In this article, two different 300 MHz bandwidth OFDM waveforms are considered, continuous and pulse version, to test suitable range/Doppler processing techniques for each case.9,10 The main characteristics and parameters of the OFDM waveforms used along the experiments are the following:

Figure 1 Long OFDM chip.
Long OFDM Chip
This waveform consists of OFDM chips that are transmitted consecutively, with cyclic prefixes (CP) inserted in between the chips as guard intervals. A chip is the basic section of the OFDM signal with a length exactly equal to 1/Δƒ. The receiver is active as long as the actual body of an OFDM chip is being transmitted, while it is turned off during the transmission of the cyclic prefix.9 The timing is depicted in Figure 1.
The carrier spacing Δƒ, the guard interval ratio α, and the numbers of carriers N were:
- N= 300000 subcarriers
- α = 0.1
- Δƒ = 1 kHz
where α is calculated as the ratio of the guard interval duration to the chip length T.9 The guard interval allows pulse compression for range processing, considering that the maximum range of interest should correspond to a delay smaller than the guard interval.

Figure 2 Short OFDM chip.
Short OFDM Chip
This waveform is typically a pulse burst, as depicted in Figure 2. Each pulse is an OFDM chip with short duration. The pulse duration T, and the numbers of carriers N were in three different cases:
- T = 1, 5, 10 µs
- PRT = 100 µs
- N = 300, 1500 and 3000 subcarriers
The pulse repetition time (PRT) values were chosen arbitrarily as for typical medium PRT radar. Nevertheless, it must be considered that the maximum desired unambiguous speed and maximum unambiguous range constrain this value.2 The pulse duration T changes according to the corresponding number of carriers inserted in each chip (T = 1 µs for N = 300, T = 5 µs for N = 1500 and T = 10 µs for N = 3000). To preserve orthogonality among carriers, the carriers spacing Δƒ must be the inverse of the chip duration T. Multiple chip durations allow different time-bandwidth products. In this case, there is no need for guard interval as will be explained later on.
As the OFDM waveform was developed for communications systems, it is important to verify the effects of electronics (deterioration of the radar performance due to hardware limitations, degradation, loss of functionality, detection capability, distortion effects) from the front-end to down-conversion to sampling, on the waveform for its performance for radar-communications fusion.

Figure 3 Anechoic chamber: map and equipment (not to scale).
Experiments and Electronic Systems Set Up
The experiments consisted of one main measurement set up, and were carried out with OFDM signals of 300 MHz bandwidth around a carrier (RF) frequency of 10.05 GHz. From the computer (PC) shown in Figure 3, the intermediate frequency (IF) complex signals are uploaded to the arbitrary waveform generator (AWG), which provides the I and Q signals with 300 MHz bandwidth on a 250 MHz carrier. For signal-conditioning, the AWG uses the differential mode, providing common mode rejection and signal fidelity.11 The AWG performs an internal sampling of the signal at 1.25 GHz. Subsequently, the I and Q signals are sent to a vector signal generator (Agilent PSG E-8267D Options 520/016) that carries out the up-conversion stage by using a local oscillator (LO) signal with a certain frequency.12 The PSG Option 520 stands for an LO frequency range of 250 kHz to 20 GHz; Option 016 allows differential wideband external I/Q inputs. Once the RF signal has passed through the transponder system, it can be visualized on an oscilloscope (DSA 91304A). The DSA samples the received signal from the transponder with a certain rate and permits downloading the received signal to the computer where it can finally be processed with MATLAB. To simulate continuous wave (CW) transmission for the long OFDM chip, the DSA is single triggered during a single measurement, whereas for the short chip case the DSA is triggered at the beginning of each pulse to simulate pulse transmission. In the latter case, it must be considered that due to the small duty cycle of this waveform it is not necessary to record the silence periods between successive pulses, and thus no guard interval, decreasing in this way the data needed to be recorded in the internal memory of the DSA.
An external down-converter system was used in front of the DSA to down-convert the RF signals to IF before sampling in the DSA (IF down-conversion). As an alternative, an RF down-conversion could be performed in MATLAB as long as coherent reception imposed for the modulation-demodulation scheme can be guaranteed for communications purposes. In the experiments campaign, it was not possible to preserve a coherent reception, thus this possibility was discarded in the bench test. The description and purpose of the external down-converter will be presented later.
The anechoic chamber and the main systems mentioned are shown in Figure 3. The DSA-AWG clock rate synchronization is carried out with a reference signal of 10 MHz, taken internally from the DSA (red arrow in Figure 3). This guarantees that the samples taken by the DSA are on the same phase values of the IF signal, and provides the same starting point of the data logging by the DSA relative to the IF signal. Synchronization between PSG and the rest of the equipment is done with a 10 MHz reference signal taken from the PSG to help in the phase-frequency stability of the LO signal when up-converting and down-converting the IF and RF signal respectively, since the same LO signal is used for both operations.

Figure 4 Transponder block diagram and signal level 1 (dBm).
Transponder and Fibre Optical Delay Line Set up
The transponder is intended for use with OFDM radar signals (see Figure 4). It was designed to possibly include variable delay and amplitude between two simulated targets. The transponder system basically consists of:
- Tx gain horn antennas: θ-3dB = 11°, 22.31 dB gain
- AWG & PSG
- 2 amplifiers: G2 (46 dB gain), G3 (20 dB gain)
- Delay line (60.18 µs): 65 dB loss, noise figure 67 dB
- Power combiner (3.5 dB loss)
- 2 variable attenuators
- 2 coaxial cables (10 meters long each): 5 dB loss each
All components are operated at least 10 dB below their 1 dB compression point to create room for the peak to average power excursions. Since it is desired to create both a single and a multi-target scenario, variable attenuators are incorporated in front of the transmitters, to possibly switch on/off one of the simulated targets and perform the difference in amplitude between the two possible targets. G2 and G3 were used to compensate for the losses of the delay line and optimize the use of its dynamic range.
The output power level setting on the PSG was -20 dBm. In the connection from the PSG output to G2 there were 20 dB losses due to the cable-connections of the anechoic chamber; thus, the levels of the signal at each point of the transponder are finally those shown in red in the figure.

Figure 5 Down-converter scheme with both LO and OFDM signal levels (dBm).
External Down-conversion System Set Up
The external down-conversion system converts the received RF signal from 10.05 GHz to an IF signal at 250 MHz. This system is shown in Figures 5 and 6. The down-converter system consists of:
- Rx gain horn antenna: θ-3dB = 11°, 22.31 dB gain
- Preamplifier: 35 dB gain
- Bandpass filter
- Double side band mixer
- Low pass filter
- 4 attenuators
- DC blocker

Figure 6 Down-conversion system, DSA, AWG and PSG.
The preamplifier is used to improve the level of the signal coming from the delay line and free space propagation. Subsequently, a bandpass filter was placed with a pass-band in the frequency range of 9.9 to 10.2 GHz. The filter presents a negligible attenuation in the band pass of interest, 15 dB attenuation for a LO feed-through at 9.8 GHz, and more than 30 dB attenuation for images in the frequency band 9.4 to 9.7 GHz. The main functionality of this filter is to suppress not only the undesired image frequencies 9.4 to 9.7 GHz coming from the I-Q unbalance of the PSG, but also the output noise of the preamplifier in the image frequency band. The bandpass filter also permits to improve the SNR by 3 dB.
The double sideband mixer is used to down-convert the RF signal to IF. For that purpose, the LO signal from the PSG is directly connected to the mixer. The mixer also converts the noise from both 9.4 to 9.7 GHz and 9.9 to 10.2 GHz bands to the 100 to 400 MHz IF output, where the noise powers add up. The mixer is surrounded by attenuators to prevent image feed back to the filters. Once the down-conversion is done, a low pass filter can be used to reduce the level of the possible undesired signals (multiples of the frequencies ± n 9.8 GHz ± m 10.05 GHz). Furthermore, to suppress the LO leakage to the sampling scope, the low pass filter can also perform a 30 dB attenuation at the LO frequency.
Finally, a DC blocker is used to eliminate the DC component of the signal towards the DSA, where the signal is delivered for visualization and analog to digital conversion. In that way, the sum of all the losses in the down-conversion component chain following the preamplifier is approximately 20 dB. The down-converter will not limit the dynamic range of the global system because the noise power output of this system is -66 dBm (16.5 dB below the noise floor of the DSA).13
Experiment Results and Discussion
Down-conversion Scheme
The external down-converter system allows for a high over sampling ratio, or the use of a lower sampling frequency, fulfilling the Nyquist-Shannon sampling theorem, since the maximum frequency of the signal is 400 MHz. If a RF down-conversion scheme in MATLAB was possible, a higher sampling frequency should be used to sample the received signal in the DSA before downloading it in the computer, since the maximum frequency of the signal is now around 10.05 GHz. Furthermore, not only the received RF signal but also the LO used to up-convert the IF waveform should be sampled, recorded and downloaded to the computer, allowing subsequently the down-conversion procedure in the computer.

Figure 7 RF 300 MHz long chip OFDM spectrum (LO = 4 GHz).
Therefore, by performing IF down-conversion, the DSA stores only the received IF signal, resulting in a lesser amount of data that has to be transferred to the computer for post processing, which also translates into a faster signal processing. The down-converter can also reduce the level of both the LO and the undesired image band coming from the I-Q unbalance of the PSG output, shown in Figure 7. To plot this figure, a LO central frequency of 4 GHz and a sampling frequency of 40 GHz were used before performing a RF down-conversion scheme realized during the test bench. Notice instead, that for IF down-conversion the PSG generates a 9.8 GHz LO signal and the DSA sampling rate is 5 GHz.

Figure 8 3000 carriers OFDM waveforms.
Distortion Effects
During the measurement campaign, distortion effects were affecting the signals. Thus, an efficient solution to correct them was done by calculating a series of coefficients to be applied in the transmitted waveform. A variation in the magnitude and phase of the output response of the internal filers of the AWG as a function of frequency is mostly responsible for the difference between the signal at the input of the AWG and the signal obtained at the input of the PSG. This variation is the result of the sine x/x (sinc) roll-off of the internal DAC and the frequency response of the reconstruction filter used for the 500 MHz channels output of the AWG.11 Therefore, the series of pre-distortion coefficients can compensate for this effect and prevent loss of functionality due to the phase modification introduced in the signal and thus a possible critical phenomenon for communication purposes.
Nevertheless, distortion effects coming from other components inserted in both the transponder and external down-conversion systems were considered (cables, bandpass filter, low pass filter, mixer). For that purpose, a 300,000 carrier OFDM signal covering the band 100 to 400 MHz, giving a finer carrier spacing of 1 kHz and random phase coding, was transmitted and received to calculate the whole system distortion effects for the operating bandwidth. This procedure was performed on the test bench, without the transponder.
Figure 8 shows in blue a received 3000 carriers OFDM chip without using pre-distortion coefficients in transmission; shown in red is the same OFDM chip, when pre-distortion coefficients are applied to the corresponding OFDM transmitted waveform.

Figure 9 Received constellations.
To verify the use of OFDM waveforms communicating radar stations, a 4-PSK constellation was used to encode two bits per symbol in the transmitted message. The received constellations are shown in Figure 9 for both cases: absence (blue) and presence (red) of pre-distortion coefficients. When no pre-distortion coefficients are used, the constellation experiences a rotation due to the variations in phase introduced mainly by the response of the AWG filters. Therefore, the effectiveness and improvements achieved through correcting the distortion feature of the global system has been proven. The residual rotation observed in the red case can be due to the distortion introduced by other extra components like the delay line, attenuators and extra cables used in the final set up, so a possible slight inaccuracy of the distortion coefficients could still be present.
To verify the radar operation, range/radial velocity detection, the ambiguity diagram obtained with a long OFDM chip is illustrated in Figure 10. The diagram is obtained with a novel signal processing technique9 for a single target case scenario. As no Doppler shift was introduced, the target appears in the zero Doppler trench. The fiber optical delay line introduces a time delay of 60.18 µs, which translates into a one-way trip distance of 18.054 km in free-space propagation conditions. The target is detected at 18.12 km, which corresponds with a time delay of 60.4 µs. The difference in the time delay is explained by the presence of the coaxial cables in the global system (transponder and down-converter) and extra cables used to perform the measurements inside the anechoic chamber. These cables introduce an extra time delay of 0.22 µs in the global system that is added to that performed by the optical delay line itself.

Figure 10 Ambiguity diagram power spectrum for one target scenario for a long chip 300,000 carriers OFDM signal.
To test the detection capability of the radar, range resolution and masking effect of close-by targets a short chip OFDM (300 carriers, time duration of 1 µs, with pre-distortion correction) was utilized in a two-target scenario. Both targets were simulated by using two identical antenna gain horns separated by two meters in range. In the anechoic chamber, the first and second gain horn were placed at 10 and 12 meters from the receiver, respectively. A 4-PSK Golay code was implemented and tested in this waveform to constrain the PAPR. The matched filter output for this waveform is shown in Figure 11. The variable attenuators determining the individual target level were set to 4 dB for both targets (blue), and 4 and 14 dB for the first and second target, respectively (red).
From the figure, both targets are easily recognized and identified according to their respective positions and levels. The x-axis has been adapted to show the match filter output around the region of interest. It should be considered that the chip OFDM waveform presents high side lobe levels at the output of the match filter, so it is necessary to apply specific signal processing techniques (out of the scope of this paper) to suppress the side lobes and allow unmasking of close-by targets with different radar cross sections.14
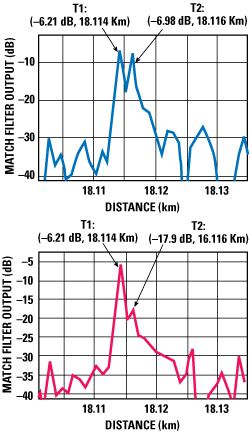
Figure 11 Two target scenario. Pulse compression output of a short chip 300 OFDM signalwith phase Golay coding.
Conclusion
A measurement campaign was carried out to demonstrate and confirm the dual use of OFDM signals for radar operation as well as for communication purposes among radar stations. OFDM signals have a large instantaneous bandwidth and also the specific coherency requirements needed for coherent radar processing imposed by the communications scheme. The experiments provided a compelling insight into the effects of the current electronic devices on the waveforms, such as amplitude and frequency distortion on the signal. Those effects were overcome to keep both the radar and communication capabilities.
The inherent high PAPR of the OFDM waveforms was limited by constraining the random phase coding, or by applying Golay codes, allowing the linear operation of the amplifiers in the receive chain and an optimal use of the dynamic range of the system.
The OFDM-radar detection capability was verified for both single- and two-target scenarios. For the multi-target scenario, the capability of coping with weak-strong targets was also shown. Recent processing techniques can be applied in the matched filter output for reduction of the side lobes level in order to unmask weak targets. Due to the flexibility of the OFDM signal, high range resolution (HRR) and frequency agility can be utilized with the aim of improving the radar operation.10 The experiments described in this article constitute a first step in the development of a new generation of radars, which can be operated in a network, using the same waveform for both radar and communications.
Acknowledgments
This project has received research funding from the Early Stage Training action in the context of the European Community's Sixth Framework Programme. The article reflects the authors' view and the European Community is not liable for any use that may be made of the information contained herein.
The measurement set up described here was made possible with the support and equipment of Agilent Technologies, MiPlaza (division of Philips Research), Philips Applied Technologies and Thales Nederland. The authors would like to thank all of them for the excellent support of these experiments.
Agilent support was kindly provided as the result of the University Innovation Award granted to some of the authors at the European Microwave Week in 2008.
References
- R.A.M. Fens, M. Ruggiano and G. Leus, "Channel Characterization Using Radar for Transmission of Communication Signals," 2008 European Wireless Technology Conference Proceedings, pp. 127-130.
- G. Lellouch and H. Nikookar, "On the Capability of a Radar Network to Support Communications," 2007 IEEE Symposium on Communications and Vehicular Technology in the Benelux Digest, pp. 1-5.
- O. Edfords, et al., "An Introduction to Orthogonal Frequency-division Multiplexing," Luella University of Technology, Div. of Signal Processing, September 1996, www.teleamerica.net/reference/Science/IntroToOrthogonalFreqDivisionMultiplexing.pdf.
- Y. Li and G. Stüber, Orthogonal Frequency Division Multiplexing for Wireless Communications, Springer, Berlin, 2006.
- R. Prasad, OFDM for Wireless Communications Systems, Artech House, Norwood, MA, 2004.
- H. Schulze and C. Lüders, Theory and Applications of OFDM and CDMA, Wideband Wireless Communications, John Wiley and Sons Ltd., Chichester, UK, 2005.
- J.G. Proakis, Digital Communications, Fourth Edition, McGraw-Hill, New York, NY, 2001.
- R.F. Tigrek and P. van Genderen, "A Golay Code-based Approach to Reduction of the PAPR and Its Consequence for the Data Throughput," 2007 European Radar Conference Digest, pp. 146-149.
- R.F. Tigrek, W.J.A. de Heij and P. van Genderen, "Multi-carrier radar Waveform Scheme for Range and Doppler," 2009 IEEE Radar Conference Digest, pp. 1-5.
- G. Lellouch, P. Tran, R. Pribic and P. van Genderen, "OFDM Waveforms for Frequency Agility and Opportunities for Doppler Processing in Radar," 2008 IEEE Radar Conference Proceedings, pp. 1-6.
- Agilent Technologies, "Agilent N8241A/N8242A Arbitrary Waveform Generators," 2006, http://cp.literature.agilent.com/litweb/pdf/N8241-90001.pdf12; Agilent Technologies, "Agilent E8257D/67D PSG Signal Generators, User's Guide," 2004-2008, www.photonics.umd.edu/umd/manuals/E8257D/users-guide.pdf.
- Agilent Technologies, "Agilent Infiniium DSA/DSO 90000A Series Data Sheet," http://cp.literature.agilent.com/litweb/pdf/5989-7819EN.pdf.
- M. Ruggiano, E. Stolp and P. van Genderen, "Resolution and Unmasking of CLEAN and LMMSE Algorithms Using Coded Waveforms," 2008 International Conference on Radar Digest, Adelaide, SA, pp. 592-597.
