MIMO radar is best for search not for track. When an array is used to search a large scan angle, it is best for maximum search energy efficiency to use subarrays of the array as the elements of the MIMO array to form what we call a subarray-MIMO or SA-MIMO. When searching out a small scan angle, the subarrays should be sized so that the volume of space illuminated by the subarrays of the subarray-MIMO array matches, or is smaller than, the volume of space to be searched. Using subarray-MIMO reduces the computation throughput. Monostatic MIMO does not provide an order of magnitude better rms angle resolution and accuracy, it is only at best a factor of 1/ better (29 percent) for a monostatic MIMO system. Thinned conventional arrays can resolve about the same number of targets and provide approximately the same resolution and angle accuracy as thinned MIMO arrays.
better (29 percent) for a monostatic MIMO system. Thinned conventional arrays can resolve about the same number of targets and provide approximately the same resolution and angle accuracy as thinned MIMO arrays.
The Multiple Input and Multiple Output (MIMO) radar has been built up as a technique that provides one or more orders of magnitude with better resolution and accuracy than conventional arrays. This is only because they have been doing an apples and oranges comparison. When doing an apples and apples comparison, the angle accuracy advantage is typically only a small amount better, approximately 29 percent. A 29 percent reduction in the angle accuracy can be realized, for example, when a linear array of N elements, or subarrays, is used as a monostatic MIMO array rather than a monostatic conventional array. Alternately, a monostatic MIMO array radar can offer the advantage of the same accuracy as a conventional array radar with a small aperture size, one that is 1/ = 0.707 smaller, or equivalently 29 percent smaller.
= 0.707 smaller, or equivalently 29 percent smaller.
The advantage of a possibly smaller antenna would be important where the antenna size is a driving factor. This advantage of better angle accuracy or smaller antenna size for a monostatic array of N elements comes at the need for ≥N times as much pulse compression match filtering and beamforming as needed for a conventional array. This large number of pulse compression comes about for two reasons. First, N orthogonal waveforms are transmitted simultaneously for the MIMO system requiring N matched filters per receive array element. Second, orthogonal or diverse1,2 waveforms are usually Doppler intolerant and hence require a bank of matched filters, let us assume a factor F more, to cover the Doppler band of the received signals of one orthogonal or diverse waveform. When the matched filters are Doppler variant then we will need FN times more beamforming as well as pulse compression. In contrast, for conventional radar, only one matched filter is needed at the output of each receiver beamformer output. Furthermore, if a chirp waveform were used, as is often done, we have Doppler tolerance for the matched filters and only one matched filter is needed for the whole band of Doppler shifted chirp waveforms instead of a bank of F of them.
MIMO is not generally efficient for track, only for search. It is not efficient for track because, typically, it illuminates a large volume of space, when one only wants to illuminate the target. Even with search, care must be taken not to illuminate a volume larger than the volume to be searched. For a given array, ideally, it should be used as a MIMO array when doing search and as a conventional array when doing track. Consider a monostatic non-thinned array used for transmit and receive. As a MIMO array doing search, it will provide approximately 29 percent better rms accuracy of the detected targets location than a conventional array. For track, to get maximum SNR and minimize the energy used for track, the array should be used as a conventional array, with its beam focused using the whole array, unless doing track-while-scan.
Generally it would not be efficient to use the individual elements of an array as the MIMO elements, even if we wanted to search out nearly the whole volume illuminated by a single element. This is because typically a single element has a power gain pattern versus scan angle θ given by cosnθ where the ideality factor n≈1.0 to 1.5. Thus to search targets at the θ= 60° for n = 1.5 we would be at the same time radiating 1/(cos1.560°)2= 8 times the energy needed to search out the target at boresite or θ= 0°. This is not efficient. We would have to radiate the energy needed to detect the target at 60° and radiate excess energy at angles for which θ ≤ 60°. What we would like to do is vary the energy used with scan angle. This can be done by not using the individual elements of the array to form the MIMO elements. Instead, we should use groups of elements of the array to form what we call subarrays. These subarrays can be made equal in size and they would all point in the same direction. By using subarrays as elements of the MIMO array, we are covering a more limited angle for a given coherent dwell. A number of dwells are used to search out the whole volume of space. As a result, we can adjust the energy radiated according to the amount needed for each dwell and be efficient for search. If the volume of space to be searched is small, so that only one dwell is needed, then for maximum search efficiency, the subarray volume of space illuminated by the subarrays should be matched to the size of the region to be searched.
By way of example, if we wanted to generate a 120° wide horizon search fence that was only one beamwidth high in height, then the subarrays would have a vertical height that produces an elevation beamwidth equal to the horizon fence elevation beamwidth. The width of the subarrays would be a few element columns wide so as to cover only a small part of the 120°, like approximately 10° wide on boresight, so that the energy radiated for each subarray scan angle could be efficiently matched to the subarray scan angle. We will call a MIMO array formed using subarrays of the array elements a subarray-MIMO (SA-MIMO) array and one that uses the individual elements an element-MIMO (E-MIMO) array. For the above horizon fence, SA-MIMO array to search out a 120° horizon fence, the energy utilized is lower than if an E-MIMO was used. Not only is SA-MIMO search more efficient than an E-MIMO, it requires much less computation. With Q subarrays, the number of matched filters and beams to be processed is (Q/N)2 lower. For N = 100 versus Q = 10, we have a factor of 100 fewer matched filters and beams. Hence the bottom line is that for an array:
- For search, SA-MIMO processing should be used.
- For track, conventional array processing should be used for maximum energy efficiency and to reduce the signal processing requirements.
If one could develop an element whose gain versus angle was constant over the search coverage angle and zero outside of this angle, then one could efficiently do search by use of the individual elements of the array for the MIMO array instead of subarrays. (This assumes the range is independent of scan angle). One way to possibly obtain such an element is to use a dome antenna.3 Besides the question of realizability of such an element using the dome antenna, the dome antenna has its issues: it is bulky, has a polarization that varies with scan angle and has losses if implemented with a passive dome lens.
A very useful near term application for MIMO is the cohering of array faces of a multifaced array system (like the AEGIS or SPY-3 systems), or for cohering two identical radars in close proximity.4-6 For the latter application, we get the increased sensitivity using existing radars without the need to develop a new radar. These two applications provide a 9 dB Power-Aperture-Gain (PAG) sensitivity advantage and 6 dB Power-Aperture (PA) search advantage, if coherent combining on transmit and receive is used. It is the one application for which MIMO can provide an order of magnitude improvement in angle accuracy. This results when the antennas for the two radars are slightly separated so that the phase centers for the two apertures result in an interferometric antenna pattern. Coherent combining can be applied to more than two radars. The combining can also be incoherent, thus not requiring coherence on transmit.7 Incoherent combining provides an 8.7 dB improvement for a Swerling 2 target.
MIMO has already been practically used for wireless communication systems, to provide increased data rate for a given bandwidth.8,9 As the cost of signal processing gets lower, MIMO should find applications for radar systems in the future.

Figure 1 Bistatic MIMO (after Frazer et al., Radarcon 2007, Boston, MA).2
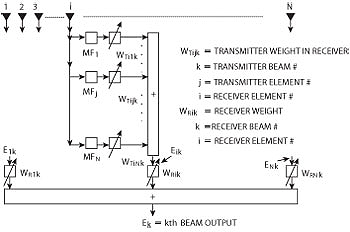
Figure 2 MIMO monostatic array receive beamformer.
First, What is a MIMO Radar System?
Consider a system consisting of linear transmit and receive antenna arrays of respectively M and N elements as shown in Figure 1 for a bistatic system.2 For a conventional radar system, all the radiating elements of the transmit antenna radiate the same waveform. Also, the antenna beams are typically focused on transmit and receive to form narrow beams at given scan angles. In contrast, for a MIMO system, generally each element of the transmit array radiates a different waveform with these waveforms often being orthogonal or diverse.1,2 As a result, on transmit, the beam energy is not focused but instead illuminates the volume of space covered by one element of the MIMO array. The M orthogonal signals go out to the target and the M echoes are received by each of the N receiver antenna elements. Hence the name Multiple Input and Multiple Output (MIMO) system. Each receive element uses M matched filters to pulse compress each of the M echoes it receives from the target. For each receive element, the matched filter outputs are provided a phase shift that focuses the transmit beam on the target. Thus for a MIMO system, the transmit beamforming is done in the receiver. These M focused transmit beam outputs of each receive element that are focused on the target are now combined to form a receive beam pointing and focused on the target. The volume of space covered and to be searched by one MIMO element is covered by forming multiple such transmitted and received focused beams that cover the volume of space illuminated by each MIMO element. As discussed, the MIMO elements could be individual elements of the array or subarrays. It is important to point out that although the MIMO transmit beams are focused in the receiver, the power density on the target is 1/M times smaller for the MIMO array of M elements than for the conventional array of M elements. This is because although we focus the transmit beam on receive, on transmit the MIMO array energy is spread out over the MIMO element field-of-view which is M times that of the conventional array. Figure 2 gives the detailed block diagram of a monostatic MIMO radar system.
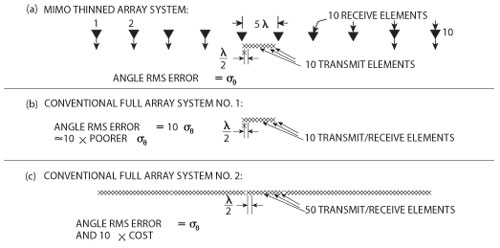
Figure 3 MIMO vs. conventional monostatic radar systems.
The purpose here is to provide a better understanding of MIMO radars and give an indication of where it is deemed useful. We will indicate where apples and oranges comparisons have been made. We will point out the issues with MIMO. Some of these issues have been pointed out before.10-14 But one still sees a wrong comparison being made.15

Figure 4 Conventional monostatic thinned array antenna patterns.
Re Better Angle Resolution and Accuracy
The claim is made that MIMO provides a resolution and angle accuracy, orders of magnitude better than with conventional array radars.1 This is because MIMO arrays which are thinned arrays can be used, with these being equivalent to full conventional arrays. Because the MIMO array is thinned, it can be longer and thus give better angle resolution and accuracy than a conventional array having the same number of elements, which they show to be a full array to avoid grating lobe. When this claim is made they are doing an apples to oranges comparison. For example, for the MIMO system, they would assume a receiver linear array (R) having 10 elements, with these elements 5λ apart, so that the array is 50λ long (see Figure 3). The transmitter linear array (T) is assumed to be a linear array of 10 elements aligned along the same axis as the receive array, R, but with its elements λ/2 apart for a total length of 5λ (Figure 3a). The transmitting array could be located at the center of the receiving array as shown. This MIMO T/R array is equivalent to an array, called MIMO Virtual Array, obtained as the convolution of the transmit and receive antenna weightings.1 Hence, for the assumption of a uniform weighting, for the T and R arrays the equivalent array is a full linear array having uniform weighting that is 50λ long (see Figure 3c).1 As a result, we have a beamwidth of approximately λ/(50λ) = 1/50 radians and an angle accuracy that is a fraction of that, depending on the SNR. For the conventional array, to avoid grating lobes, they assume a non-thinned 10 element array having a λ/2 spacing, so its length is 5λ. This array is used for transmit and receive (see Figure 3b). Its beamwidth is λ/(5λ) or approximately 1/5 radians, ten times wider than for the MIMO array, so its beamwidth and accuracy are ten times worse. They also point out that to get the same resolution with a conventional array having no grating lobes, you would need an antenna consisting of 50 elements (see Figure 3c,) which would be approximately 50 times more expensive than the MIMO thinned array of Figure 3a. This is true, but they are making the wrong comparison. They should be using the same two T and R arrays for the conventional system as used for the MIMO system. The receive array will then have a main lobe and several grating lobes, but these grating lobes occur at the nulls of the transmitting array, if uniform illumination is being assumed as we are assuming here (see Figure 4a). We are then left with a two-way pattern with just the main lobe, whose width is approximately 1/50 radians, which will give us an angle resolution and accuracy for the thinned conventional system that is about the same as for the MIMO array and it will not have grating lobes (see Figure 4b). So, in conclusion, we can get the same resolution and accuracy with an antenna system consisting of a conventional thinned receive array and full transmit array just as we can with an equivalent MIMO when an apples to apples comparison is being made. (Care needs to be used in forming the MIMO Virtual Array by the convolution of the thinned and full array weightings. Specifically the thinned array needs to have 50 what are called “dummy elements” at the λ/2 spacing between the active receive elements; see Appendix online at
www.mwjournal.com/MIMOappendix.)
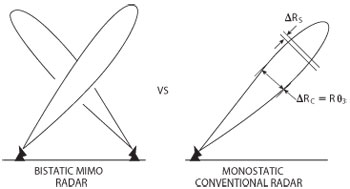
Figure 5 Bistatic MIMO vs. conventional monostatic system: Apples and oranges comparison.

Figure 6 Bistatic MIMO vs. bistatic conventional systems: Proper comparison.
A second example, where they do an apples to oranges comparison, is the following. For the MIMO system, they assume two radars that are very widely spaced and compare it to the performance obtained when just one of these radars is used as a monostatic radar (see Figure 5). For the widely spaced MIMO systems, one will get better detection, because of the angle diversity offered with the bistatic MIMO system and also much better target position accuracy than with the monostatic radar. Again this is the wrong comparison. They should be comparing the bistatic MIMO system with a conventional bistatic radar system having the same spacing between the radars. Then, one would be getting a position accuracy and detection performance about the same as for the MIMO system, because we realize the same angle diversity for the equivalent identical bistatic conventional system. Figure 6 shows the advantage offered by the conventional bistatic radar system. Here, there are nearly orthogonal range measurements for the conventional radar. It is also possible to use the interferometric lobes, obtained by the coherent combining of the outputs of the two conventional bistatic radars, just as well as the MIMO system can. Though the interferometer lobes are very narrow for many geometries, there are then too many in one beam width. This is the case illustrated in Figure 7. The interferometric lobes here are only 1 µr wide, which becomes 0.02 m at a range of 20 km. The number of the interferometric lobes in the main lobe beamwidth of 10 mr is approximately 10,000. It will be difficult to determine which interferometric lobe the target is located in, to make use of the fine accuracy offered by the interferometric lobes. This is true for both the conventional and MIMO bistatic radars.
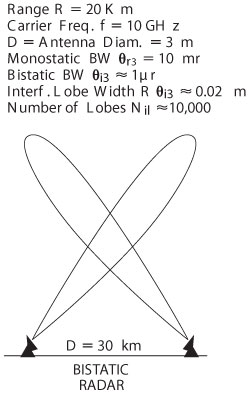
Figure 7 Bistatic MIMO and bistatic conventional interferometric lobes.
Let us go back to our example in Figure 3b. Consider the linear array consisting of 10 elements λ/2 apart having a length of 5λ. Assume it is used for transmit and receive. It would be useful to compare the angle accuracy we can get, when used as a conventional array with the accuracy obtained when it is used as a MIMO array. A simple back-of-envelope analysis tells us that the MIMO array will have a better angle accuracy, but it is only approximately  better or, equivalently, the rms angle error is approximately 29 percent smaller.16 This advantage occurs because, for the MIMO array, we get angle error estimates from the transmit as well as the receive array, whereas, for the conventional array, we get the angle estimate only from the receive array (see Appendix online at www.mwjournal.com/MIMOappendix). The extra signal processing load to do MIMO processing to get the better angular accuracy versus that for the conventional array is large and will not always be warranted at present.
better or, equivalently, the rms angle error is approximately 29 percent smaller.16 This advantage occurs because, for the MIMO array, we get angle error estimates from the transmit as well as the receive array, whereas, for the conventional array, we get the angle estimate only from the receive array (see Appendix online at www.mwjournal.com/MIMOappendix). The extra signal processing load to do MIMO processing to get the better angular accuracy versus that for the conventional array is large and will not always be warranted at present.
Search vs. Track
As pointed out previously, MIMO is efficient for search, not for track. This is because with the MIMO radar, one is illuminating the whole volume that a single MIMO element illuminates. With all the elements assumed identical, they all illuminate the same volume of space. If there is only one target in the volume, most of that energy is wasted. What is wanted for track is a beam focused on the target, with this focused beam formed from the whole array.
It is important to emphasize that the improvement in angle accuracy of the factor of  is realized for a monostatic full MIMO array system, while doing search of a volume of space. For a linear MIMO monostatic array of N elements, on receive, N focused beams are simultaneously formed that cover the volume of space illuminated by one element. One can think of it as doing track-while-scan. This is one application for which MIMO is well suited. There are situations where this type of search is desired for a conventional system. One such case is where one wants to search out a volume of space, using many receive beams simultaneously, with the volume of space illuminated by one spoiled transmit beam.
is realized for a monostatic full MIMO array system, while doing search of a volume of space. For a linear MIMO monostatic array of N elements, on receive, N focused beams are simultaneously formed that cover the volume of space illuminated by one element. One can think of it as doing track-while-scan. This is one application for which MIMO is well suited. There are situations where this type of search is desired for a conventional system. One such case is where one wants to search out a volume of space, using many receive beams simultaneously, with the volume of space illuminated by one spoiled transmit beam.
The Multi-function Phased Array Radar (MPAR) system, being developed by Lincoln Laboratory as the prime and M/A-COM as the subcontractor with the funding coming from the FAA, has one such mode.17 MPAR is an effort to develop the next generation air traffic control and national weather surveillance services. Having the search mode for the MPAR is desirable in order to search out the volume of space in a short time to keep the occupancy for this search down. Otherwise, one runs out of time. For the MPAR system on receive, 24 beams are generated, consisting of two rows of 12 beams, one above the other to simultaneous search out quickly the volume of space covered by these 24 beams. A spoiled transmit beam is used to illuminate this volume of space on transmit. The 24 receive beams are formed by combining the outputs of the array subarrays. The spoiled transmit beam is formed using the whole array with the spoiling being achieved with possibly a quadratic phase applied across the array. The MPAR uses overlapped subarrays on receive, to avoid generating severe grating lobes in the process of generating these multiple beams from the subarrays.
For a MIMO array system doing the MPAR type search, the receive MIMO array subarray size would be adjusted such that the subarray element size produces a beam that just covers the volume of space to be searched. The overlapped subarrays for the MPAR have approximately a sin x/x illumination, which in turn generates approximately a rectangular subarray beam pattern, which in turn eliminates the generation of severe grating lobes as mentioned previously. For a MIMO system to do the MPAR type of search, using the array subarrays as the elements, it would also be desirable to use overlapped subarrays on transmit, so as to just illuminate the volume to be searched. This is a little more difficult to achieve on transmit for MIMO array. Instead one would use non-overlapped subarrays on transmit that cover the volume of space to be searched.
In the future, when digital beam- forming at the element level is cost effective, the volume of space that the subarray MIMO array elements illuminate could be made adaptable. The subarrays would be made to illuminate the volume of the 24 beams to be searched out, or any other number of beams. On receive, 24 or any other number of beams, could be formed just like in the conventional MPAR system. Using MIMO, we would realize the  improvement in angle accuracy in search over the conventional MPAR system. Also, on receive, these subarrays would be overlapped to avoid grating lobes being formed for the 24 or so receive beams. Right now, the hardware and processing cost required do not appear to warrant the use of MIMO to get this
improvement in angle accuracy in search over the conventional MPAR system. Also, on receive, these subarrays would be overlapped to avoid grating lobes being formed for the 24 or so receive beams. Right now, the hardware and processing cost required do not appear to warrant the use of MIMO to get this  improvement.
improvement.
An alternate conventional system approach for illuminating the volume of space to be searched, other than using a spoiled transmit beam, as done for the above MPAR type system, is to use what is called “machine gunning.” With machine gunning, a focused beam is formed on transmit that sequentially illuminates each of the 24 receive beam positions, using pulses that immediately follow one another. This is done before the echoes from any of the pulses are received. After the transmission of the 24 pulses, there is a receive listening period for the echoes. With this approach it is possible to use a different carrier frequency for the beams to avoid interference of the signals between beams. The advantage of this approach, over beam spoiling, is that the coherence time needed for each of the pulses is 1/H, which is required with beam spoiling, where in the MPAR example, H = 24. Apparently the longer coherence time required by the MPAR system with the use of beam spoiling is not a problem. The H times longer coherence time is needed with the use of beam spoiling, because with the beam spoiling the gain of the antenna on transmit is 1/H times lower than without the spoiling as done with machine gunning.
As indicated in the introduction, the use of the array subarrays for an MPAR MIMO type system, that is SA-MIMO, is better for the efficient use of search energy over using the elements of the array, that is E-MIMO. As pointed out earlier, the 120° horizon fence search example requires more energy to do the search with E-MIMO than with SA-MIMO.
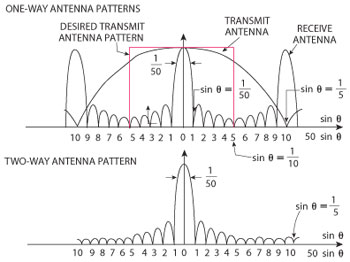
Figure 8 Conventional monostatic thinned array antenna patterns: Ideal transmit pattern.
At this point, it is worth going back to the thinned array example of Figure 3a. We showed that the conventional radar, having the thinned receive array and full transmit array, can have approximately the same resolution and accuracy as that of the equivalent MIMO array. This comparison was somewhat unfair, because the MIMO array would be achieving the desired performance for all angles in an element’s coverage which, as indicated previously, would be typically approximately 120° for an embedded element gain given by cosnθ with n = 1 to 1.5. For the conventional system, we are illuminating the more limited angle of Δμ±1/10 in u space where μ= sin θ (see Figure 4). This is an angle of +5.7°. Hence, to cover the whole volume with the conventional thinned array, we need to have dwell illuminations that cover the other angles which are needed to cover the 120° scan volume. This is achieved by scanning the transmitter to illuminate these other scan angles during these other dwell times. This is desirable, since we can then adjust the energy needed for the search, dependant on the off-boresite angle, as done for the subarray-MIMO discussed previously. In Figure 4, we show only one focused receive beam covering the azimuth angle Δμ=1/50 at boresite. To cover the angle illumination by the transmit beam of Δμ±1/10, we need to generate simultaneously ten more focused receive beams. These non-boresight beams, however, will have grating lobes, the boresite receive antenna pattern grating lobes at μ= ± 1/5 in Figure 4 no longer being at the nulls of the transmit pattern. We can eliminate these grating lobes by using a two to three times larger transmit array that has approximately a sin x/x illumination across the transmit antenna, so as to produce an approximately rectangular transmit antenna beam pattern (see Figure 8). Such a rectangular beam pattern would eliminate or nearly eliminate the grating lobes when the transmit beam is pointed at boresite and for all other transmitter scan angles. This solution requires different power levels for the transmit elements for this example but that is not a problem and the power amplifiers can all be operated at saturation (Class C for maximum efficiency). The conventional thinned array will be approximately 3.7 dB more efficient in searching out a 120° horizon fence, than the E-MIMO thinned array; for an ideality factor of n = 1, about 5.2 dB for n = 1.5. We will call the conventional thinned array given by Figure 3a with the transmit antenna extended two to three times the modified-conventional-thinned-array.
Another possible way to achieve a rectangular element pattern for the conventional and MIMO arrays is to use elements that have a nearly rectangular antenna pattern. This could possibly be done with the rod element,18,19 if one only needed to cover the angle covered by the 3 dB transmit beamwidth of Figure 4. The rod element has approximately a rectangular antenna pattern.
Because our conventional thinned array system of Figure 3a has the same resolution as its MIMO equivalent system, it will be able to resolve as many targets occurring simultaneously in the coverage region, as does its MIMO equivalent and the MIMO equivalent full array of Figure 3c. Because it has the same resolution, it will also be able to estimate the parameters of these targets just as well as its MIMO equivalent system does. This is contrary to the results given1, Sect. 1.3 for thinned MIMO array systems. The reason for this difference is that for the conventional array, a single element is assumed for the transmit antenna and a full array of N elements for the receive antenna. This is the wrong combination to use for the comparison. In our comparison to achieve equivalence, we use a full transmit array of M elements, equal to about 20, to achieve the nearly rectangular transmit beam pattern shown in Figure 8. For receive we use a thinned array identical to the MIMO thinned array.
If the thinned array example given only had to cover a horizon fence 2 sin-1 0.1 = 11.5° wide at boresite, the modified-conventional-thinned-array would be approximately 10 dB more efficient than the E-MIMO thinned array when n = 1, approximately 9 dB for n = 1.5.
Issue of Coherence Time Needed With MIMO VS. Conventional Array
Often, it is indicated that an N element MIMO array has the disadvantage of requiring N times the coherence integration time as needed when using a conventional array. Here again an apples to oranges comparison is being made. This comparison is only true when comparing a MIMO system of N antenna elements with a conventional array system using the same array of N array elements, but with either: (1) the conventional array is searching out the whole volume of space sequentially with one transmit and receive focused beam; or else, (2) the conventional array is searching the volume of space with multiple focused beams, N, being formed simultaneously on receive and machine gunning being used on transmit. If instead for the conventional system beam spoiling is used, transmit as done with the Lincoln Laboratory MPAR, the coherent integration time for the conventional MPAR type system is the same as the equivalent MIMO system. Searching the volume of space with a conventional system using one transmit and receive beam is not efficient. It requires N times the search time that is needed with machine gunning on transmit or with beam spoiling on transmit and using in both cases N simultaneous receive beams.
Processing Complexity Accrued With MIMO
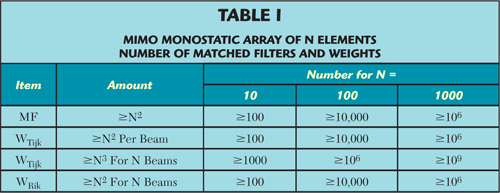
Assume a monostatic full (that is non-thinned) array of N elements. With MIMO, the whole volume would be searched out by forming N focused transmit beams that cover the volume of space to be searched.22 These beams would be formed in the receiver. Each of the N receiver elements will have N matched filters (MF), (see Figure 2 and Table 1). These N matched filters are needed to process the N orthogonal or diverse waveforms transmitted from the N transmitter elements. Since there are N such receiver elements with each having N MFs, N2 matched filters are needed for the pulse compression in the MIMO system of one focused transmit beam. The N2 MFs used to form the kth beam are also shared to form the other k = 1 to N transmit beams. Thus to form the N transmit beams N2 MFs are needed. Following the N2 MFs for the kth beam formation are the weights WTijk that contain the phase shifts needed to focus the kth transmit beam in the direction of the kth beam. For the N2 MFs outputs for the kth beam, N2 weights are needed, because i = 1 to N and j = 1 to N for a given kth beam, the receiver elements being indexed as “i” and the transmit waveform from the “jth” transmit element being indexed as “j”. To form the kth transmit beam the receiver weight outputs are combined for the ith element to form the output Eik. This is done for the other elements to form Eik, i = 1 to N (see Figure 2). These outputs are in turn combined with the weights WRik, i = 1 to N, to form the kth receive focused beam output Ek (see Figure 2). A total of N2 + N weights WTijk and WRik are thus used to form the kth focused receive beam or approximately N2 for N »1. Finally for the N receive beams, a total of N3 + N2 such weights are thus needed or ~N3 for N »1. The transmit and receive beams are identical (overlapping in space) here for this monostatic MIMO system, where the same array is used for both transmit and receive. Hence the indexing k is identical for the receive and transmit beams. Because k = 1 to N there are a total of N2 transmit beams that are formed. Finally N receive beams are formed. Thus for the MIMO system N2 + N beams are formed to generate the N receive beams (see Table 2). The above discussion did not take into account Doppler variance. Recall that with Doppler variance a bank of F Doppler MF for each orthogonal or diverse waveform is required and as a result F times more MFs and weights would be needed.

Let us compare the SA-MIMO with a conventional radar regarding the computation needed. For both we shall assume N subarrays of the same size. We shall assume that the space to be searched is equal to the volume illuminated by one subarray, however, for the conventional array we shall form the transmit beam by using the whole array with beam spoiling. On receive for both we are covering the whole volume of space using N simultaneous receive focused beams as done by the SA-MIMO array. This would give a fair comparison. For the conventional radar, only N MFs are needed, versus FN2 for the SA-MIMO radar, a factor of FN more for the SA-MIMO radar. For the conventional radar only N2+N weights are needed, N on transmit to form the one spoiled beam and N2 on receive to form the N focused receive beams. For the SA-MIMO, we need FN3+FN2 weights, a factor of FN more. For N = 100 we are talking about F times 10,000 MFs for the SA-MIMO radar versus 100 for the conventional radar, using a chirp waveform. There are cases where linear FM waveforms can be used for the MIMO system, like for OTH systems.2,20 The ≥ in Figures 9 and 10 is to allow for the possibility of Doppler intolerance for the MIMO radar.
Nulling Out Interference
The advantage of the MIMO is that it can adaptively put nulls in the transmit beam as well as in the receive beams where there is clutter. Assume again a MIMO linear full array of N elements used for both transmit and receive. It was indicated previously that N2 + N weights are needed to form the one beam. Thus an N2 + N by N2 + N matrix has to be inverted to form one beam adaptively. For N = 1000 we need to invert approximately a million by a million array. For N = 100 we need to invert a 10,000 by 10,000 element array. In either case, this is a lot of computations and we have not taken into account Doppler intolerance. For a conventional array, we can easily achieve the same result. Specifically, we can put nulls in the transmit beam in the direction of clutter if we have a clutter map or if we know in advance where the clutter is. For example in a ground based or ship based radar we know the clutter is at the horizon so we put a null in the antenna transmit pattern as well as in the receive pattern for the sidelobes on the horizon. Thus we do not always need MIMO to put nulls in the transmit pattern. The extra degrees of freedom (DOF) the MIMO brings come at a high computation cost. Right now, we are having a problem in our systems handling the DOF we have with our conventional arrays. We cannot do the adaptive nulling at the element level or even at the subarray level in many applications. It is too costly in computations. Li and Himed consider the use of transmit subarrays for MIMO, TS-MIMO, to reduce the computation load for putting nulls in the direction of interference.24

Figure 9 Coherently combine N radars: (Bob Enzmann/George Thome & Leon Green patents4-6; see also Coutts & Coumo, ieee sam 2006, Boston21 and Brookner patent7).
Where MIMO is Useful at Present and the Near Term
Two radars in close proximity are useful for cohering on transmit and receive (see Figure 9).4-6,21 It provides a 9 dB improvement in PAG or equivalently SNR for a target being detected or tracked. For search, it provides a 6 dB advantage in PA. This is not without a cost. It requires that during the time of the combining, both radars be used for tracking one target or for searching the same volume of space. This is the one application that can provide an order of magnitude improvement in angle accuracy. MIT Lincoln Laboratory has demonstrated the combining in real time of dish radars using MIMO.21,22
Brookner et al. describes a patented incoherent MIMO technique for combining two radars.7 It uses different carrier frequencies for the two radars. This technique has the advantage of providing an 8.7 dB improvement in sensitivity for a Swerling 2 target for a 90 percent detection. This improvement is realized by choosing the carrier frequencies far enough apart to provide independence of the echo amplitude returns for the two frequencies. For a Swirling 1 target, the frequency diversity then lowers the target fluctuation loss to the point of almost making up for the video integration loss.
It would be useful for monostatic radar applications where the size of the antenna must be small and a 29 percent reduction in antenna size would be important. No doubt MIMO will find practical uses in the future as signal processing continues to get less expensive. Another near term potential candidate for MIMO is the OTH radar. MIT Lincoln Laboratory and the Australians have been doing work in this area.20,22,23 OTH looks like a good application, because there are not too many elements and the bandwidth is small so the processing is not excessive. Figure 1 shows the architecture for a bistatic MIMO system which applies for an OTH system where the transmit and receive antennas are usually separated.
Acknowledgment
The author would like to thank Mike Sarcione and Jian Wang and Dr. Jama Mohamed, all at Raytheon Co., for reading and making suggestions for the paper. Brookner would also like to thank Professor Jian Li (University of Florida) and Dr. Daniel Zwillinger (Raytheon) for their input.
References
- J. Li and P. Stoica (editors), MIMO Radar Signal Processing, John Wiley & Sons Inc., Somerset, NJ, 2009.
- G. Frazer, Y. Abramovich and B. Johnson, “Spatially Waveform Diverse Radar: Perspectives for HF OTHR,” Proceedings of the 2007 IEEE Radar Conference, Boston, MA.
- E. Brookner, Radar Technology, Artech House, Norwood, MA, 1977.
- G.D. Thome, R.P. Enzman and F. Steudel, “System and Method for Coherently Combining Plurality of Radars,” US Patent Application Publication, dated October 5, 2006, Pub. No. US2006/0220951 A1.
- G.D. Thome, R.P. Enzmann and F. Steudel, “System and Method For Coherently Combining Plurality of Radars,” US Patent No. US 7,358,892 B2, dated April 15, 2008.
- L. Green, “Cooperative Radar System,” US Patent No. US 6,362, 774 B1, dated March 26, 2002.
- E. Brookner, D.V. Manoogian and F. Steudel, “Multiple Radar Combining for Increased Range, Radar Sensitivity and Angle Accuracy,” US Patent No. US 2005/0231420 A1, dated October 20, 2005.
- L. Bai and J. Choi, Low Complexity MIMO Detection, Springer, New York, NY, 2012.
- D. Tse and P. Viswanath, Fundamentals of Wireless Communications, Cambridge University Press, New York, NY, 2005.
- E. Brookner, “Breakthroughs in Phased Arrays and Radar,” Nanyang Technological University, Singapore, October 11, 2006.
- E. Brookner, “Breakthroughs in Phased Arrays and Radar,” 2006 CIE International Conference on Radar, Shanghai, China.
- E. Brookner, “Breakthroughs in Phased Arrays and Radar,” Fourth IEEE Workshop on Sensors Array and Multichannel Processing(SAM-2006), Waltham, MA, July 12-14, 2006.
- E. Brookner, “Latest Developments in Phased Arrays, Radars, Technology and Processing,” 5th Military Radar Summit, February 6-8, 2012, Washington D.C.
- F. Daum and J. Huang, “MIMO Radar: Snake Oil or Good Idea?,” IEEE A&E Systems Magazine, 2009.
- MIMO papers at EuMW 2011.
- D. Bliss, MIT Lincoln Laboratory, private communication.
- J. Herd et al., MPAR, IEEE Radar-2010, Washington D.C.
- S. Skobelev, “Flat Topped Patterns,” 1996 IEEE International Symposium on Phased Array Systems and Technology Digest, pp. 438-443.
- S. Skobelev, Phased Array Antennas with Optimized Element Patterns, Artech House, Norwood, MA, 2011.
- G.J. Frazer, Y.I. Abramovich, and B.A. Johnson, “MIMO Radar Limitations in Clutter,” RadarCon-2009, Pasadena, CA.
- Coutts and Coumo, “Radar Combining,” IEEE SAM 2006, Boston, MA.
- F. Robey et al., “MIMO Radar Theory and Experimental Results,” Proceedings of the 2004 Asilomar Conference, pp. 300-304.
- G.J. Frazer, Y.I. Abramovich, B.A. Johnson and F.C. Robey, “Recent Results in MIMO Over-the-Horizon Radar,” IEEE Array-2010.
- H. Li, and B. Himed, “Transmit Subaperturing for MIMO Radars with Co-Located Antennas,” IEEE Journal of Selected Topics in Signal Processing, February 2010, pp. 55-65.
- E. Brookner, Practical Phased Array Antenna Systems, Artech House, 1991.
- E. Brookner, Aspects of Modern Radar, Artech House, 1988.
