PIM IN HIGH POWER, MULTI-CARRIER SYSTEMS

Depending upon the operating frequency and application, the choice of connector head can be vital to achieve good performance. For cellular installations with two carrier frequencies and high signal levels, PIM can occur from the mixing of these two signals in the transmission line. PIM can also occur in the passive components used in multi-carrier systems, including the circulators, duplexers, attenuators, waveguide and antennas. Although the nonlinear intermodulation distortion (IMD) products from PIM are generally at low signal levels, the resulting PIM can degrade the dynamic range of the system - unacceptably in highly sensitive radios, where PIM can interfere with the transmit and receive bands of a communications link.

There are two main sources of PIM:

• Electrothermally induced PIM (ET-PIM), occurring at a rough surface or a metal junction.
• Paramagnetic or ferromagnetic materials.

Other sources, such as tunneling and nonlinear conductivity, can exacerbate the problem of PIM but are not known as major contributors.

With ET-PIM, any metallic surface is a resistive element whose resistance is a function of its temperature and temperature coefficient of resistance (TCR), per the thermo-resistance equation. A thermal and electrical interdependence occurs from the dissipation of electrical power causing self-heating. This can be described by its thermal capacity, the ability of a material to store heat, and the rate at which the heat is radiated into the surrounding environment when the temperature increases. These self-heating effects behave dynamically with a periodically varying resistance, especially when two or more high frequency signals are applied and with a nonlinear metal contact, such as inadequate mating or surface roughness. The combination of thermal resistance and capacitance represents a lowpass filter in the thermal domain. If the beat frequency of the two carriers falls within this thermally created lowpass filter, the periodic heating and cooling of the resistive element functions as a passive electrothermal mixer, up-converting the envelope frequencies at baseband to RF frequencies and creating PIM.^2-3

ET-PIM has a strong link to current density, the TCR and the electrical and thermal conductivities of the metals used in the connector heads (see Table 1). A low TCR and high thermal conductivity material with moderate current densities will yield lower PIM. Current density increases at high frequency due to the skin effect; this is worse with dimensionally thinner coax, as the current density increases as the radial distance to the center conductor decreases. The uniformity of the current along the face of a metal also gets worse with increasing surface roughness. Microscopic inconsistencies on a metallic surface can limit the amount of current flowing through the junction, adding to poor PIM performance. A wider connector head would likely be better, with an adequate tightening of the joints.

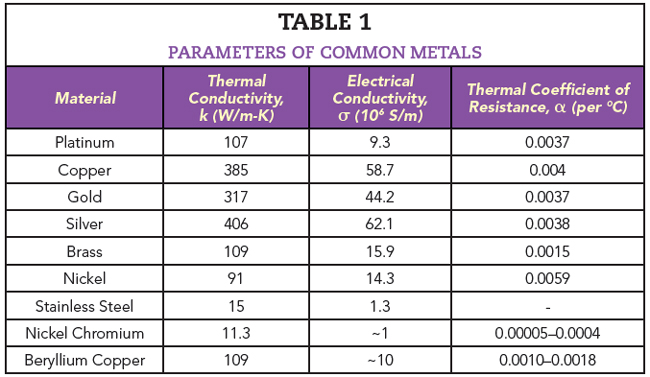
Using magnetic materials is typically a secondary contributor to PIM in sensitive cellular systems. Here, PIM is caused by the magnetic hysteresis of ferromagnetic materials or the irreversible magnetization of the ferromagnetic material due to an externally applied alternative magnetic field. Nickel and chromium are common ferromagnetic materials used in coaxial connector heads. This source of PIM can be effectively eliminated by carefully selecting the base and plating materials in the connector head, such as a plated brass body. In some cases, nickel-chromium components function better than platinum non-ferromagnetic components; this could reflect the improved TCR of nickel-chromium compared to platinum.⁴

TEMPERATURE EFFECTS

Amplitude Variation

Coaxial cables will physically expand with increasing temperature, and these variations cause measurable differences in the insertion loss and phase. The increase in loss is due to the decrease in conductivity of the metallic material with temperature: as the temperature increases, molecular vibrations increase the collisions of the electrons traveling through the medium. This phenomenon is commonly seen with highly conductive materials, since the thermal conductivity increases with average particle velocity, while electrical conductivity decreases due to the vibrations inhibiting the forward movement of charge, as described by the Wiedemann-Franz law. In general, the resistance of pure metals increases linearly with temperature.

The resulting increase in insertion loss with increasing temperature is generally unavoidable; however, phase instability can be optimized by carefully selecting materials.

Phase Stable Coax

Phase instabilities are a consequence of the change in the electrical length, i.e., the number of wavelengths for a length of cable. For most systems, this is not a concern; however, for systems relying on phase for constructive or destructive interference - beam steering, for example - repeatable and stable phase and amplitude over temperature and cable flexing is vitally important.

Phase stability is a measure of the ability of the insertion phase (∠S₂₁) of a coaxial cable to remain constant with temperature change and mechanical stressors such as flexure, vibration or bending. The time delay and insertion phase are related by

where f is the frequency; τ the time delay, usually measured in nanoseconds; and∠S₂₁ the insertion phase, measured in degrees. The relationship between time delay and the length and relative permittivity of a coaxial cable is given by

where l is the mechanical length of the coax. Both l and ε_r change with temperature. While the increase in length at high temperatures generally opposes the decrease in dielectric constant, they are not typically in proportion to yield stable time delay or phase.

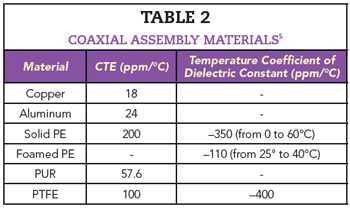
As the coax will experience linear volumetric expansion at elevated temperatures and contraction at cooler temperatures, the linear coefficient of thermal expansion (CTE) is a useful parameter for understanding the incremental increase and decrease in the size of a solid material with respect to temperature - as it will be directly proportional to the increase or decrease in the cable. Table 2 shows the CTE of some materials used in coaxial assemblies and the temperature coefficients of the dielectric constant, showing the insulating materials expand more rapidly than the metals. The cable length is fixed by the conductors, due to their inherent stiffness, while the elastic modulus of metals are typically in the hundreds of gigapascals (GPa) and insulators rarely go beyond 5 GPa. Therefore, the rapidly expanding insulating material becomes compressed between the inner and outer conductors. This compression is more pronounced at lower temperatures, where the contraction of the shielding causes a density increase in the dielectric that can ultimately change the dielectric constant, depending on the type of material used. The dielectric also affects the electrical length of the cable, from the change in the dielectric constant over temperature. As stated earlier, foamed dielectric materials tend to be more stable with temperature changes.

The applications for a phase stable cable often require more than one cable to distribute system signals. In such cases, the phase change among the coax cables in a set must be as close as possible. Skew-matched cable assemblies ensure a tight phase match between cables, in terms of length and ε_r, despite temperature changes or flexure. Phase tracking - close matching of phase - between coaxial assemblies is also important. Preconditioning through a controlled temperature cycling of the cables can be performed to provide temperature stress relief, so the cables will perform reliably in harsh climates. This process anneals the dielectric and metallic conductors, reducing the likelihood of surface cracks and internal stressors that could cause premature failure. In addition to temperature variation, phase instability often occurs from the flexure at the point of the bend.

MECHANICAL STRAIN

Vibrations, Bends and Flexure

Mechanical strain can have a significant impact on electrical performance. Cables may be subject to vibrations from wind shear or frequent flexure caused by the application. Both the connector heads and coax can undergo a combination of tensile, compressive, bending, shear and torsional forces. Of all these, coaxial strain from frequent bending or flexure is particularly troublesome, as it increases the strain on the connector-cable junction and the shielding. Referring to equation 2, the forces on the shielding material are far greater than those on the center conductor and dielectric, and the elastic modulus of the jacketing and dielectric polymers are several orders of magnitude lower than those of the metallic conductors in the shielding and center conductor, making them far less susceptible to bending stresses. Since the coax is orthotropic and symmetric, designed for impedance and continuity, the neutral axis would most likely be along the very center of the center conductor. Therefore, the center conductor will typically experience less mechanical strain due to bending than will the shielding. For this reason, flexible coaxial cables should have a combination of these qualities:

• Smaller inner and outer conductor diameters, for less overall bend strain.
• Non-metallic layers between bonded aluminum foil, braiding and jacketing materials to lower the coefficient of friction during rubbing.
• Stranded center conductor to distribute bend stresses between wires.
• Strain relief boots or overmolding.
• Armoring to prevent bends beyond the prespecified bend radius.

These attributes will mitigate the strain at the bend in a coax and yield better phase performance. Almost any bend in a coax will cause a bend at the joint between the connector head and cable, which can cause the relatively elastic cable to push against the stiff crimp joint, eventually kinking the cable or breaking through the jacketing material. Strain relief boots are nearly always necessary in assemblies that experience high flexure.

Impact, Crushing and Abrasion

Coaxial cables can experience shear forces due to crushing or torsion from installation or daily use. Typically, a cable can withstand forces from a human step; however, the strain from a rodent gnawing or from the weight of a vehicle could cause enough pressure to deform the cable and cause failure. Typically, basic abrasion and tear resistance are ensured by using a strong jacketing material such as polyurethane (PUR). Additional crush resistance is enabled through armoring; often an interlocked metallic hose can provide extraordinary immunity to crushing.

SUMMARY

The design of a coaxial cable requires careful consideration of its application, as the cable will likely not function properly without appropriate definition. Low loss, phase stable cables often require the use of relatively low dielectric constant materials and extensive thermal cycling. Cables that are expected to withstand bending and flexure will likely be smaller in diameter or have external features to prevent failure, such as armoring or strain relief boots. Low loss coaxial cables for cellular installations are typically thicker than their RG counterparts and have low PIM connector heads that do not use ferromagnetic metals. Every application calls for a slight adjustment in cable fabrication to achieve long operational lifetimes and optimized electrical performance.

References

1. Rodríguez-Pérez, M.a., et al. “The Effect of Cell Size on the Physical Properties of Crosslinked Closed Cell Polyethylene Foams Produced by a High Pressure Nitrogen Solution Process,” Cellular Polymers, Vol. 21, No. 3, 2002, pp. 165–194., doi:10.1177/026248930202100302.
2. Wilkerson, J.r., et al. “Electro-Thermal Theory of Intermodulation Distortion in Lossy Microwave Components,” IEEE Transactions on Microwave Theory and Techniques, Vol. 56, No. 12, 2008, pp. 2717–2725., doi:10.1109/tmtt.2008.2007084.
3. J. R. Wilkerson, I. M. Kilgore, K. G. Gard and M. B. Steer, “Passive Intermodulation Distortion in Antennas,” IEEE Transactions on Antennas and Propagation, Vol. 63, No. 2, pp. 474–482, February 2015.
4. J. R. Wilkerson, K. G. Gard and M. B. Steer, “Electro-Thermal Passive Intermodulation Distortion in Microwave Attenuators,” 2006 European Microwave Conference, Manchester, 2006, pp. 157–160.
5. G. Rodriguez, “Phase Stability of Typical Navy Radio Frequency Coaxial Cables,” U.S. Naval Applied Science Laboratory, web: apps.dtic.mil/dtic/tr/fulltext/u2/628682.pdf.