SIMULATION OF INTERDIGITATED STRUCTURES USING TWO-COUPLED-LINE MODELS
Abstract:
Some commercial microwave CAD programs do not incorporate models for more than three coupled lines. This fact is especially critical in the design and analysis of multiple-line planar structures, such as interdigital, combline and hairpin filters, meander lines, spiral inductors and couplers. This article presents a new, simple technique to simulate circuits with multiple coupled lines in commercial simulators without suitable models. The negative transmission line (NTL) method uses pairs of two coupled lines, single transmission lines, current-controlled current sources (CCCS) and voltage-controlled voltage sources (VCVS), which are available in any commercial microwave CAD program. One of the greatest advantages of the proposed technique is that the analysis and optimization parameters are the physical dimensions of the coupled structure (line widths and spacings); therefore, optimization and tuning of the structure are easy and fast.
Accurate models for the analysis of multiconductor structures are not available in some commercial microwave CAD programs. Therefore, the analysis and simulation of multiple-line planar structures such as interdigital, combline and hairpin filters, meander lines, spiral inductors and couplers using these types of simulators require new methods. To overcome this limitation, two alternative approaches have been proposed. One approach uses an equivalent circuit composed of ideal noncoupled transmission lines and short-circuited stubs.1–4 (This technique does not include effects such as losses in the substrate and conductors, discontinuities and dispersive effects of the transmission lines, which are very important in the case of microstrip lines.) The other approach consists of modeling the multiple-line structure by decomposing it into pairs of coupled lines.5–7 This method is based on the identities developed by Grayzel,8 which divide every line into several parts to form pairs of coupled lines. Using this technique, every coupling can be simulated with the model of two coupled lines, which is available in any microwave CAD tool. In most cases, dispersive effects and substrate and conductor losses are not considered.6,7 However, Denig's approach5 takes them into account. The main disadvantages of this technique are that it is only valid for coupled lines of equal width and the optimization and tuning process is very tedious because the structure parameters are even- and odd-mode impedances that need to be calculated every time a geometrical modification in the coupled structure is performed. Additionally, a coupled structure whose even- and odd-mode impedances do not have a physical realization can be obtained.
The proposed technique utilizes pairs of coupled lines and solves the problems with Denig's method. The physical dimensions of the coupled structure are used as parameters so the optimization process is easier and faster and the physical perspective of the design is not lost. Using this technique, n coupled lines (which can be of different widths) are transformed into (n – 1) pairs of coupled lines and (n – 2) single transmission lines loaded by an element referred to as a negator. This element can be implemented by CCCSs and VCVSs. As in Denig's approach, the proposed technique does not take into account coupling between nonadjacent lines.

Fig. 1 A planar structure of three coupled lines.
Fig. 2 The noncoupled equivalent circuit for three coupled lines.
PROCEDURE FUNDAMENTALS
The method described in this article is based on the noncoupled equivalent circuit for coupled lines proposed by Sato and Cristal,1 where the multiple-coupled-transmission-line structure is transformed into a set of noncoupled transmission lines. Figure 1 shows the physical parameters for three coupled lines where W1, W2 and W3 are the widths of the lines and S12 and S23 are the spacings between lines 1 and 2 and 2 and 3, respectively. The structure length is l. Sato and Cristal's equivalent circuit for the three-coupled-line case is shown in Figure 2. The coils represent short-circuited stubs. The length of all of these lines is the same as the multiple-line structure, and the characteristic admittances Yii and Yij are elements of its characteristic admittance matrix. Lines of characteristic admittances –Y12 and –Y23 are NTLs.
If a line of characteristic admittance –Y22 of the same length is introduced, as shown in Figure 3, as well as another line of characteristic admittance Y22 in parallel, an equivalent network is obtained. However, in this network, the coupling between lines 1 and 2 can be separated from the coupling between lines 2 and 3. In this way, the coupled structure has been decomposed into two independent couplings plus an NTL.
NTL SIMULATION
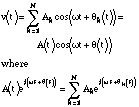
The ABCD matrix of a lossless NTL of characteristic impedance –Z0 (Z0 > 0) is represented as
where
q = electrical length
The matrix of Equation 1 can be obtained by multiplying both sides of the ABCD matrix of the transmission line Z0 as

Then, Equation 2 can be seen as the resultant ABCD matrix of three quadripoles connected in cascade where the central matrix corresponds to a transmission line of impedance Z0. The first and last matrices correspond to the elements called negators. An equivalent circuit for a possible implementation of the negator is shown in Figure 4. This component can be implemented by a CCCS and VCVS. Therefore, an NTL can be replaced by an identical line with positive characteristic impedance and two negators.
GENERALIZATION OF THE TECHNIQUE
Using the negators, the three-coupled-line network ends up as shown in Figure 5. A line of characteristic admittance, Y22, and two negators appear instead of the previous NTL. It can be proved that the geometric parameters of the independent couplings are the same as the physical parameters of the original structure after performing two approximations: The width of the line i, Wi, depends only on the characteristic admittance of this line, Yii. In addition, the spacing between lines i and i + 1, Si,i+1, depends only on the characteristic admittances of these lines (Yii and Yi+1,i+1) and the mutual characteristic admittance Yi,i+1. As a result, the separated coupling between lines 1 and 2 can be characterized by the width of lines 1 and 2 of the original structure and by the physical spacing between them. The same can be said of the separated coupling between lines 2 and 3. The width of the single transmission line Y22 will be the width of line 2.
The generalization to the n-coupled-line case is shown in Figure 6, where the negators have been included. The shaded area corresponds to the couplings between adjacent lines. Every single line, together with its two negators, comprises an NTL. As stated previously, the parameters of this network are the physical parameters of the coupled structure. The pairs of coupled lines and the single transmission lines can be simulated using the corresponding models of the simulator. Since these models include losses, the NTL method is capable of estimating them.
SIMULATIONS AND MEASUREMENTS

Fig. 3 Coupling separation in a three-coupled-line structure.

Fig. 4 A negator circuit representation.

Fig. 5 A three-coupled-line structure using negators.

Fig. 6 The generalized structure for n coupled lines.
In order to test the utility and validity of the proposed technique, an example of a microstrip tapped-line interdigital filter is presented. It is a fifth-order Chebyshev filter with 0.01 dB of ripple and a passband from 4.5 to 5.5 GHz. The plastic substrate chosen was 0.025"-thick epsilom-10 with a dielectric constant of 9.9. This filter has been designed using the method proposed by Caspi and Adelman.9 Table 1 lists the calculated filter parameters. The layout and schematic of the filter are shown in Figures 7 and 8, respectively. Although it has been removed from these figures for clarity, the ground connection of the resonators has been modeled by an inductance of 0.05 nH.
|
TABLE I | ||||
|
Strip |
Zsuboe (W) |
Zsuboo (W) |
W (mm) |
S (mm) |
|
1 and 2 |
35.36 |
26.00 |
1.400 |
0.425 |
|
2 and 3 |
34.25 |
27.62 |
1.400 |
0.675 |
|
3 and 4 |
34.25 |
27.62 |
1.400 |
0.675 |
|
4 and 5 |
35.36 |
26.00 |
1.400 |
0.425 |
|
Tap point (1sub2) |
|
2.125 mm |
| |
|
1 sub1 + 1 sub 2 |
|
4.825 mm |
| |
|
1a |
|
0.140 mm |
| |

Fig. 7 The filter layout.

Fig. 8 The filter's implementation using the developed technique.

Fig. 9 A practical implementation of the negator using the LIBRA series IV simulator.

Fig. 10 Simulation vs. measurements of the filter's transmission characteristics.
If the filter is simulated using the n-coupled-line generalization shown previously, convergence problems arise, which can be overcome with a small resistor placed as shown in Figure 9. A value of 10–3 W has been used in the simulation so that its presence does not affect the simulation results.
The simulations are performed using the HP EEsof LIBRA Series IV simulator, and the results are compared with Ansoft's SERENADE 7.5 simulator, which is a CAD tool providing a full-wave model for multiple coupled lines. The LIBRA circuit file used to simulate the filter is shown in Appendix A. The NTL method has also been tested using SERENADE to show its dependence on the two-coupled-line model used. Additionally, a comparison with the measurements is made.
Figure 10 shows the transmission behavior of the filter predicted by the NTL method in LIBRA compared with the response of the full-wave model of SERENADE. In the filter passband, the agreement between SERENADE and the measurements is excellent because the full-wave model is very accurate. Since the NTL model is approximate, it provides a good estimation of the bandwidth and passband losses with a small frequency shift (approximately 100 MHz upwards) of the passband. However, the disagreement with the measurements increases around the filter edges. The main reason is that the NTL method does not consider coupling between nonadjacent lines. Even though the SERENADE model does not have this limitation, it cannot adjust very well for the filter edges either.

Fig. 11 Simulated vs. measured input reflection characteristics.

Fig. 12 Transmission behavior simulation comparison using the full-wave model and NTL method in SERENADE and the NTL method in LIBRA.

Fig. 13 Reflection behavior simulation.
With regard to the reflection behavior, shown in Figure 11, the deviations of the NTL model are larger - up to 10 dB more optimistic. The difference is not due to the NTL model itself but the two-coupled-line model used by LIBRA.
If the NTL method is implemented in SERENADE, the response in the passband is closer to the full-wave model, as shown in Figures 12 and 13. This result illustrates the dependence of the NTL method on the two-coupled-line model used. Around the filter edges, the behavior of the NTL method in SERENADE is very close to that in LIBRA because the NTL method does not consider the coupling between nonadjacent lines.
CONCLUSION
A new technique that allows simulation of multiple-coupled-transmission-line structures using commercial microwave CAD simulators without suitable models has been demonstrated. The coupled structure is decomposed into a cascade of two-coupled-line and single-transmission-line sections loaded by negators. Every negator can be implemented using a CCCS or VCVS. This method uses the physical dimensions as analysis and optimization parameters. For that reason, the physical aspect of the structure is always accounted for. Optimization and tuning are easier and faster than with previous techniques. The new method provides a good estimate of the behavior of the coupled structure that is useful in the design stage.
ACKNOWLEDGMENT
This work was supported by contract P96093259 of INDRA DTD and Project TIC-99-1172-C02-01 of the National Board of Scientific and Technology Research (CIYCIT).
References
1. R. Sato and E.G. Cristal, "Simplified Analysis of Coupled Transmission-line Networks," IEEE Transactions on Microwave Theory and Techniques, Vol. 18, No. 3, March 1970, pp. 122–131.
2. G. Matthaei, L. Young and E.M.T. Jones, Microwave Filters, Impedance-matching Networks and Coupling Structures, McGraw-Hill, New York, 1964.
3. J.A.G. Malherbe, Microwave Transmission Line Filters, Artech House, Norwood, MA, 1979.
4. E.G. Cristal, "Tapped-line Coupled Transmission Lines with Applications to Interdigital and Combline Filters," IEEE Transactions on Microwave Theory and Techniques, Vol. 23, No. 12, December 1975, pp. 1007–1012.
5. C. Denig, "Using Microwave CAD Programs to Analyze Microstrip Interdigital Filters," Microwave Journal, Vol. 32, No. 3, March 1989, pp. 147–152.
6. M.A. Larson, "A New Element-saving Equivalent Circuit for the Analysis of General Coupled n-Wire Transmission Lines," IEEE Transactions on Microwave Theory and Techniques, Vol. 39, No. 11, April 1988, pp. 1855–1861.
7. G. Mazzarela, "CAD Modeling of Interdigital Structures," IEEE Transactions on Education, Vol. 42, No. 1, February 1999, pp. 81–87.
8. A.I. Grayzel, "A Useful Identity for the Analysis of a Class of Coupled Transmission-line Structures," IEEE Transactions on Microwave Theory and Techniques, Vol. 22, October 1974, pp. 904–906.
9 S. Caspi and J. Adelman, "Design of Combline and Interdigital Filters with Tapped-line Input," IEEE Transactions on Microwave Theory and Techniques, Vol. 36, No. 4, April 1988, pp. 759–763.


