Figure 1 The aperture tuned antenna system consists of a matching circuit, simulated two-port antenna and aperture tuner.
In the quest for larger bandwidths and throughput in mobile communication, operators are looking for an ever-increasing number of frequency bands, using multiple bands simultaneously with carrier aggregation techniques and adopting multi-antenna technologies. For mobile portable devices, the evolution of the industrial design toward large displays, large batteries and thin profiles has pushed antenna design requirements to the limit. Antenna tuning techniques have, therefore, become increasingly important, as it is difficult to cover all required mobile frequency bands with a fixed antenna resonator and fixed impedance matching circuit.
Besides allowing the mobile device to set the antenna to operate optimally at the desired frequency band (open loop tuning), tunable antennas also enable the implementation of “adaptive tuning,” also known as closed loop tuning. The mobile device will, in this case, continuously try to adapt the antenna to the changing environment—for example, loading by the user’s hand. As the name suggests, this technique also requires actively measuring one or more performance parameters, which the controller can use as guidance for setting the tuner control.
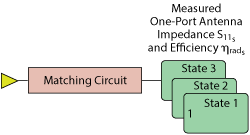
Figure 2 An alternative, measurement-based design approach for an aperture tuned antenna.
Frequency tunable antennas can be implemented in two basic methods. In impedance tuning, the matching circuit connected to the antenna feed will contain a tunable element, such as a tunable capacitor or a switch. A more sophisticated technique is aperture tuning, where a tuning network is attached to the antenna resonator using a dedicated connection located a given distance from the feed port. The latter technology has recently gained the most interest in the industry thanks to the higher potential performance benefits. However, it can be very sensitive to the losses in the tuning element, which must be able to sustain higher currents and voltages.1
Several technologies are currently available for implementing reconfigurable networks to be used as antenna tuners. Solid-state switches can be used in single-pole multi-throw configurations, which allows for the connection and disconnection of external passive components such as inductors and capacitors. Different technology variants, such as high resistivity silicon on insulator (HR-SOI)2 and silicon on sapphire,3 are continuously evolving to achieve the best figure of merit for the switch, indicated by the product of on resistance and off capacitance (RonCoff in ps). Microelectromechanical systems (MEMS) technology can potentially surpass solid-state technology in this figure of merit, but the commercial availability of MEMS switches for the RF market is still limited. Besides using a switch, a variable capacitor available as a single component can provide a more compact alternative. Digitally variable capacitors (DVC) are commercially available and largely deployed in antenna tuners in phones on the market, implemented either in solid-state or MEMS technologies.4
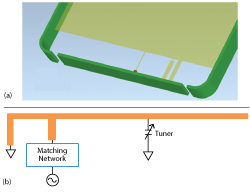
Figure 3 3D model (a) and schematic (b) of an aperture tuned antenna.
This article aims to show how to design an aperture tunable antenna for frequency band selection. A variable capacitor is used to implement the aperture tuner while a fixed matching circuit at the antenna feed is co-designed for best performance across all required frequency bands. It will be demonstrated how the co-design of the aperture tuned antenna and the fixed matching network are key for achieving optimum performance, since the operation of the aperture tuner greatly affects the impedance presented at the antenna feed port. The design flow also presents opportunities for designing antennas for carrier aggregation combinations: a given tuner configuration is set to cover a combination of bands instead of a single one. However, this significantly complicates the design effort and falls beyond the scope of this work.
DESIGN METHODOLOGY
The design goal for the aperture tuned antenna and fixed impedance matching network is to maximize the total radiation efficiency over each frequency band. This is more relevant than getting the lowest possible return loss at the feed, since any losses in the matching components or the tuner will improve the feed match, but at the expense of radiated power.5 At least as an initial design candidate, the aperture tunable antenna can be designed using simulated antenna, tuner and matching component data. As shown in Figure 1, the antenna system is a two-port system with port 1 being the feeding port and port 2 the aperture port. The design task is to design a matching circuit for port 1 and find the best possible values for the aperture tuner at port 2 to cover all the frequency bands.
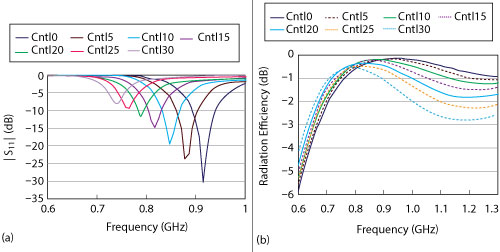
Figure 4 Simulated |S11| (a) and radiation efficiency (b) vs. tuning state.

Figure 5 Matching networks for the fixed (a) and aperture tuned (b) antenna designs covering bands 5, 8 and 17.

To describe the problem in a mathematical sense, assume that a tuner component has been attached to the aperture port 2, with value Cs for state s. Assume that the frequencies have been separated to n frequency sets Fk, with k = 1,…,n, where each set contains one of multiple frequency bands, which need to be covered with one tuner state. The total efficiency is denoted by η(f, S, ηrad, P, Cs(k)), which depends on the frequency f, the two-port antenna scattering parameters S, radiation efficiency ηrad of antenna port 1, fixed component values P of the matching circuit at port 1 and tunable component values Cs(k) in the aperture port, where s(k) is the chosen tuner state for frequency set k. The optimization task can be written as follows:
The search is for the values of the fixed matching components and the tuner states which maximize the minimum efficiency over all the frequencies in all the frequency sets, where the tuner can assume a different state for each frequency set. Note that this formula describes the optimization when the matching circuit topology is known. Instead of manually trying out different matching topologies, circuit synthesis algorithms can be used to produce multiple optimized matching circuit topologies, dramatically speeding up the design process of tunable matching circuits.

Figure 6 Simulated |S11| (a) and total efficiency (b) comparisons of the aperture tuned and fixed matching circuit designs covering bands 5, 8 and 17.
In many cases, the accurate design of the tunable system is not possible using simulations; in an actual mobile device, many small details may affect antenna performance. The electric and dielectric properties are unknown, making accurate electromagnetic simulation impossible. The radiation efficiency of the feed port will depend on the value of the aperture tuner, and this interaction is difficult to capture accurately when using circuit simulators or matching circuit optimization tools. When carrying out measurements, it is often difficult or impossible to measure the aperture port impedance accurately, due to the proximity of the feed port.
In contrast, the following single-port design flow based on a prototype, which contains the aperture tuner, is proposed (see Figure 2). The radiation efficiency (ηrads) and input impedance (S11s) of the antenna prototype is measured or simulated for each tuner state (s). This information is used in the matching circuit optimization to find the best tuner state, which varies with frequency band, and a fixed matching circuit.
When s(k) is the chosen tuner state for frequency set k, the optimization task (for a fixed matching circuit topology) can be written as:
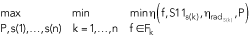
What needs to be ascertained are the values of the fixed matching components and the tuner states (the tuner is embedded in the measured/simulated one-port data) that maximize the minimum efficiency over all the frequencies in all the frequency sets, where the tuner can assume a different state for each frequency set. The optimization algorithm is searching an optimal impedance and efficiency data set (implicitly the best tuner state) for each of the frequency sets, while optimizing the fixed matching circuit values for best global performance. Both the matching circuit topology and component values can be optimized if the design tool supports topology synthesis in this context.
DESIGN EXAMPLES
This section presents an aperture tuned antenna design based on the methodology presented in the previous section. Figure 3 shows a 3D model for a metal frame phone antenna based on a planar inverted-F antenna (PIFA) and the schematic of the design concept, extended with an aperture tuning connection point (i.e., tuner). To tune the antenna across all desired low frequency bands (700 to 960 MHz), a MEMS-based DVC is connected to the antenna resonator 10 mm from the feed. The variable capacitor is digitally controlled with 5 bit resolution, with a total of 32 control states (Cntl 0 to Cntl 31). Figure 4 shows the |S11| of the antenna for a selection of tuning states, based on the antenna impedance S11s at the reference plane of the antenna feed.
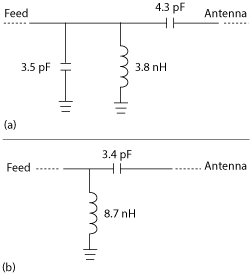
Figure 7 Matching networks for the fixed (a) and aperture tuned (b) antenna designs covering bands 5, 8 and 28.
The steps to design the aperture tuning circuit are:
- Simulate/measure the return loss and efficiency of the antenna for each state of the tuner
- Import the S-parameters and efficiency data into the matching circuit design software
- Calculate the proper tuning state and fixed matching network to cover the desired frequency bands.
The S11 and efficiency results for individual tuning states are calculated in EMPIRE XPU 3D EM simulation software.6 The co-simulation of the matching network and aperture tuned antenna calculation uses matching circuit optimization software.7 The tuner used in this design is a MEMS-based DVC.8 It has an ultra-low resistance together with high voltage handling, which is suitable for efficient antenna tuning.1,4 Realistic models of the inductors and capacitors, including component losses and parasitic reactances, were used in the example.
To show the effectiveness of the presented design methodology, two examples are presented. In both, the aperture tuned antenna will be compared to a more traditional fixed antenna, where both antenna options include an optimized fixed matching network at the feed.
Covering Bands 5, 8 and 17
The results presented here represent a typical design requirement for portable mobile devices, which includes the following frequency bands: LTE band 17, LTE band 5 (GSM850) and LTE band 8 (GSM900). This band configuration is typically quite challenging for small handset antennas. The matching network is limited to one component for the tuned antenna, while three components are required for the fixed antenna (see Figure 5). The aperture tuned antenna, while requiring an extra contact point for the tuner, requires considerably less space in feeding area due to the lower number of matching elements. Figure 6 compares the |S11| and the total efficiency for the proposed aperture tuned antenna design technique versus a fixed reference antenna for all required frequency bands, and Table 1 summarizes the efficiency results of the two solutions. The aperture tuned antenna improves the total efficiency by more than 3 dB in band 5 and more than 1.5 dB in bands 8 and 17.

Figure 8 Simulated |S11| (a) and total efficiency (b) comparisons of the aperture tuned and fixed matching circuit designs covering bands 5, 8 and 28.
Covering Bands 5, 8 and 28
A more challenging design is covering LTE band 28 with LTE band 5 (GSM850) and LTE band 8 (GSM900). The very large bandwidth of band 28 makes this band configuration extra challenging compared to the previous example. Figure 7 shows the schematic of the fixed matching network for both solutions. In this case, due to the large bandwidth requirement of band 28, the aperture tuned antenna required two matching components, while the fixed antenna matching network required three elements, as before. Figure 8 compares the |S11| and total efficiency of the two solutions, and Table 2 summarizes the total efficiency versus frequency band for each solution. The aperture tuned antenna improves the efficiency by more than 1 dB in all the desired frequency bands; in band 28, the average total efficiency is improved by 1 dB without trading off efficiency in the other two bands.
CONCLUSION
Tunable antenna solutions are increasingly being used to enhance the radio performance of mobile handheld devices. The design and implementation of tunable antennas is challenging as it involves the co-design of the tuner states and the fixed matching circuitry to obtain optimal total efficiency. This article presents a novel measurement-based design flow for aperture tunable antennas, using the measured impedances and radiation efficiencies of the antenna system for each state of the digitally controlled aperture tuner capacitor. This collection of data is used to synthesize an optimal fixed matching circuit topology at the antenna input, to optimize the discrete component values and to select the optimal tuner states.
The benefit of this approach is that the measurement setup of the antenna impedance and radiation efficiency is greatly simplified and provides more reliable data. In contrast, an accurate two-port measurement involving both the feed and the aperture tuning ports is difficult due to the proximity of the ports, influence of the aperture port measurement cable to the antenna performance and the presence of other components. Proper incorporation of the radiation efficiencies would be complicated in a two-port approach.
While electromagnetic simulation can alleviate the measurement setup problems at early design phases, the radiation efficiency problem still persists, and the approach presented generalizes to simulated data as well. The design examples demonstrate that the aperture tuning approach with ultra-low loss tuner components provides a significant improvement over the reference case utilizing just a fixed matching circuit at the antenna input—even when using fewer matching components than in the reference case.

References
- P. A. Tornatta, R. Gaddi, “Aperture Tuned Antennas for 3G-4G Applications Using MEMS Digital Variable Capacitor,” Microwave Symposium Digest (IMS), 2013 IEEE MTT-S International, June 2‐7, 2013.
- A. Botula et al., “A Thin-Film SOI 180 nm CMOS RF Switch Technology,” Silicon Monolithic Integrated Circuits in RF Systems, 2009 (SiRF ‘09), January 19‐21, 2009.
- R. Novak, “UltraCMOS® Technology for High-Performance Switch Paths and Tunable Components,” 2013 International Symposium on VLSI Technology, Systems, and Applications (VLSI-TSA), April 22-24, 2013.
- V. Joshi et al., “MEMS Solutions in RF Applications,” IEEE SOI-3D-Subthreshold Microelectronics Technology Unified Conference (S3S), October 7-10, 2013.
- J. Rahola, “Optimization of Matching Circuits for Antennas,” Proceedings of the EuCAP 2011 Conference, Rome, April 11-15, 2011.
- Empire XPU, IMST GmbH, www.empire.de/main/Empire/en/home.php.
- Optenni Lab Matching Circuit Optimization Software, www.optenni.com.
- Cavendish Kinetics, www.cavendish-kinetics.com.

