The fast growing demand for microwave communication technologies, such as broadband wireless and satellite communication, point-to-point radio links, as well as a variety of radar systems, brings up stringent requirements to modern microwave filter design techniques aimed at minimization of electromagnetic interference, cross talks and jamming. The most critical electrical requirements (such as low insertion loss, greater skirt selectivity and flat group delay), applied to the front-end filters and having a direct effect on the RF system performance, create a traditional, straightforward way of filter design not always acceptable. In a number of cases, the physical dimensions, weight and cost also become an essential factor driving the design approach. Today, when the highly competitive business environment allows little time for the design and development of high performance filters, the knowledge and understanding of “nontraditional” filter configurations, based on nonadjacent resonator coupling, can be a crucial contributing factor to a successful project completion.
The subject of this article is a discussion of the concept and understanding of cross-coupling as a multipath signal propagation through the filtering media with stress on the physical aspects rather than mathematical formulation. A number of solutions to the coupling configurations are offered, based on both experimental and software simulation results. A complex evaluation of these configurations with regard to their electrical performance, high power handling capabilities, practical realization and cost factor is also given. Special attention is given to equalized group delay filters with enhanced skirt selectivity as a more sophisticated requirement for the telecommunications industry. Some useful software recommendations are also provided.
Basic Cross-coupling Configurations
A block diagram of the widely used four-element filter with one capacitive cross-coupling is shown in Figure 1. Assuming a combline filter structure, the coupling between sequential resonators is magnetic, which means that the phase of the main path signal measured at the center frequency at the top of the first and last resonator is 270° apart. The minimal signal coupled through the capacitive coupling element maintains the same 270° phase shift, so both main path and cross-coupled signals are in phase at the center frequency at the output resonator.

Fig. 1 A four-element filter with one capacitive cross-coupling.
Since the slope of the phase versus frequency for the main (four resonators) path is significantly steeper than the one for the cross-coupled (two resonators) path, if considered separately (see Figure 2), the out-of-phase condition is met right outside the filter passband formed by the main path. The superposition of two compatible out-of-phase signals at the filter output results in their mutual cancellation, which produces two reject notches placed on the skirt and greatly improves the near passband selectivity (see Figure 3). It means that two real frequency (jω-axis) transmission zeroes1,2 are incorporated into the transmission function. The location on the skirt and the depth of the reject notches depends on the cross-coupling value. A greater cross-coupling implies a broader passband response of the virtual two-element filter and, consequently, its phase slope is flattening. The out-of-phase condition is met at frequencies closer to the main path passband, so the reject notches move toward each other, making the passband response look more square. At the same time, a greater cross-coupling allows a larger part of the signal skipping two inside resonators, resulting in an amplitude imbalance between two paths at the notch frequencies, a lesser depth of the notches and a degraded out-of-band rejection. Two responses with weaker and stronger coupling are illustrated in the figure.
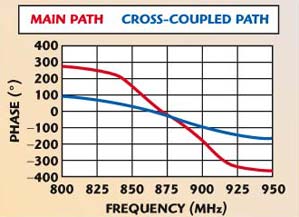
Fig. 2 Phase responses of the main and coupled paths.

Fig. 3 Four-element capacitive cross-coupling, as examples of greater and weaker coupling.
Replacing a capacitive cross-coupling with an inductive one changes the coupled path phase shift from 270° to 90°, which makes the out-of-phase condition meet at the center frequency. It means that a pair of transmission zeroes is placed on the real (σ) frequency axis.1,2 Figure 4 illustrates a flat group delay response resulting from an inductive cross-coupling at the expense of the skirt selectivity.

Fig. 4 Four-element, 1-4 inductive cross-coupling with flat group delay response.
The cross-coupling examples discussed above, skipping an even number of elements (particularly two, so-called quadruplet, in this case), have a quasi-symmetrical effect to the main path of consecutively coupled resonators response, simply because the in-phase or out-of-phase conditions are met at the center frequency or outside the passband at frequencies approximately equally distanced from the center. An asymmetry in the response can be introduced by the cross-coupling skipping an odd number (for simplicity sake — one, forming a so-called triplet) of elements, as shown in Figure 5. Applying the same argumentation, the phase shift between cross and main path signals is ±90° at the center frequency, for inductive or capacitive cross-couplings, which with the difference in phase slope for both paths, results in the out-of-phase condition being met at the high or low side of the passband skirt correspondingly (see Figures 6 and 7).

Fig. 5 Four-element filter with a triplet cross-coupling.

Fig. 6 Three-element, 1-3 inductive cross-coupling.

Fig. 7 Three-element, 1-3 capacitive cross-coupling.
The basic cross-couplings discussed represent a solution for many bandpass filters. They could be incorporated as a part of a more complex filter, in a variety of configurations, in order to enhance its properties.3 A typical example of this approach would be the cascading of the triplets or quadruplets allowed by a meandered filter configuration. The physical effect of skipping a greater number of elements or multiple cross-coupling is based on the same in-phase/out-of-phase signal superposition approach, but results in a more complicated effect on the filter response.
Cross-coupling in Folded Filters
More complicated cross-coupling configurations, involving a greater number of elements skipped as well as multiple cross-couplings, are used to optimize the filter response and satisfy a variety of sometimes mutually exclusive requirements, such as broader passband, flatter group delay/insertion loss with sharper skirt, etc. A few more practical configurations based on a so-called folded filter structure1,2,4,5 will be considered. First, a six-element combline (inductively coupled resonators) filter with single capacitive cross-coupling connecting the first and sixth elements is shown in Figure 8. The two signal paths, main and cross-coupled, maintain out-of-phase conditions at the center frequency, producing a pair of real axis (equalization) transmission zeroes, while the second out-of-phase propagation occurs outside of the passband, which results in two additional real frequency (jω-axis) transmission zeroes with corresponding rejection notches placed on the skirts. Due to the significant phase slope difference between the two paths, the in-phase signal summation occurs at the passband edges and does not have any effect on the overall response.

Fig. 8 Six-element filter with a single capacitive cross-coupling.
The practical significance of such a cross-coupling configuration is that two parameters such as improved selectivity and flattened group delay can be maintained simultaneously. In other words, a pair of real (σ-axis) equalization transmission zeroes and a pair of real frequency (jω-axis) transmission zeroes at the skirts are obtained with a single cross-coupling. Adding a second, minimal inductive cross-coupling between the second and fifth elements slightly improves the selectivity and broadens the flat group delay response. A six-element combline type filter response is shown in Figure 9. The amplitude and group delay responses are resulting from single and double cross-couplings as described. It should be mentioned that, if a flatter group delay is the goal, the rejection notches location and, consequently, the near passband skirt selectivity will be predetermined. A further increase of the capacitive cross-coupling between the first and sixth elements results in the amplitude response squaring (the reject notches move toward each other) and a reversed (over-coupled) group delay response, which makes possible the use of such a filter, similar to a non-minimum phase all pass network, as a separate equalizer in cascade with a given circuit. An inductive element, connecting the first to the sixth elements, does not have any positive effect on the response, simply because the in-phase condition occurs at the center frequency and at the skirts, which degrades the selectivity while an out-of-phase signal occurs inside the passband, close to the band edges and does not improve the group delay response. The situation changes if the first to sixth inductive element provides a minimal complementary coupling to the stronger second to fifth (widely used, quadruple) capacitive or inductive coupling. In the first case, the addition of two real frequency (jω-axis) transmission zeroes outside of these caused by a capacitive cross-coupling may significantly improve the out-of-band rejection. Figure 10 illustrates the amplitude response of two six-element filters with single (quadruple) and dual cross-couplings. In the second case, the flattened group delay may allow for more than 80 percent of the design band. A certain out-of-band amplitude response asymmetry (transmission zeroes location) is caused by the frequency dependent coupling elements. Table 1 provides a comparative analysis of the six-element general section (folded configuration) part of the bandpass filter having a combination of typical even-element (two and four) skipped cross-couplings.

Fig. 9 Six-element filter with (a) 1-6 capacitive cross-coupling only and 1-6 capacitive and 2.5 inductive cross-coupling, and (b) stronger 1-6 capacitive cross-coupling and reversed group delay response.

Fig. 10 Six-element filter with (a) single capacitive 2-5 and dual 2-5 capacitive and 1-6 inductive cross-couplings, and (b) with two 1-6 and 2-5 inductive cross-couplings.

The approach utilizing cross-coupling loops one inside another (nested), skipping an even number of resonators, has a great practical application because it allows the use of the so-called folded filter configuration (sometimes called canonical) with easy realizable capacitive or inductive cross-couplings between electrically nonconsecutive but adjacently located resonators.3,6 Figure 11 shows the software simulated response of a 12-element moderate band (five percent) filter with four cross-couplings. Based on the folded configuration concept and employing 2-11, 3-10, 4-9 elements capacitive and 5-8 elements inductive cross-couplings, the filter allows a flattened group delay for approximately 70 percent of the passband with enhanced skirt selectivity. It should be mentioned that the passband insertion loss is directly related to the group delay and exhibits flatness within the same range inside the passband.

Fig. 11 Twelve-element flat group delay filter.
Asymmetry in the Filters with Cross-couplings and Methods of Its Compensation
Asymmetry in the filters, in general, may be conditioned through a variety of factors. Associated with the cut-off frequency, the propagation through the waveguide predetermines an asymmetry in the waveguide bandpass filter (steeper low side skirt of the passband). A predominantly magnetic coupling in combline filters, associated with the electrical length of the TEM resonators, contributes to enhanced selectivity of the high side skirt. For frequencies above the passband, where TEM resonators maintain approximately 90° electrical length, combline structures theoretically provide no coupling at all. At the same time, a second passband and an infinitely increasing attenuation at lower frequencies may also have an effect on the symmetry of specific microwave filter responses. More generally, for lumped element L-C type bandpass filters, which are often used to simulate microwave filter passband responses, the symmetry is determined by the number of transmission zeroes at infinity and DC.7,8 Cross-couplings skipping an even number of elements in the bandpass filters usually aggravate the natural asymmetry of the passband response due to multipath signal propagation imposed, which in turn causes in-phase/out-of-phase conditions allocated asymmetrically with respect to the passband. As was demonstrated previously, the software-generated responses for the filters with cross-couplings skipping an even number of elements exhibit an asymmetry, which takes the form of slightly different high/low side skirt selectivity, which is directly related to the location and depth of the transmission zeroes as well as a skewed passband group delay related to the same fact. The addition of a minuscule cross-coupling skipping an odd number of elements (sometimes called diagonal) as well as a slight frequency off-setting of the resonators inside the cross-coupling loop may be a solution to the passband asymmetry problem when required. The synthesis and network extraction-based software used for filter design (the last section will provide a brief overview of the software available) usually computes a so-called coupling matrix, which is intended for perfectly symmetric responses. The guided wave technology9 synthesized coupling matrix for a six-element flat group delay filter is shown in Table 2.

The incorporation of minimal diagonal cross-couplings between the second and sixth (capacitive, three elements skipped), and third and fifth (inductive, one element skipped), as well as a slight fourth resonator frequency off-set, results in perfectly symmetric theoretical responses and further flattened group delay.
Practical Realizations of the Filters with Cross-couplings
Practical implementation or, in other words, the technology employed in order to realize microwave bandpass filters (that is, type of resonators, transmission lines) with numerous cross-couplings is determined by a number of factors, such as insertion loss, overall dimensions, power handling and spurious response or high side out-of-band rejection. At that point, the choice of the transmission lines or resonators is not different than for the traditional filter design approach. The implementation of the cross-coupling is much more dependant on transmission line and resonator type utilized for a given filter.
Waveguide-type folded filter configurations described in the literature,11–14,16,17 employing single- or dual-mode waveguide cavity resonators, allow both capacitive and inductive coupling between electrically non-adjacent resonators, making possible the variety of the responses previously mentioned. In recent years, waveguide filters based on circular resonators and employing two orthogonal TE111 modes12,14 (thus providing two resonances per single cavity) have been widely used. A two-resonator (four-element) section of such filters (quadruplet) with a single cross-coupling is well described in the literature.10 The proper positioning of the tuning screws on both resonators may deliver an inductive cross-coupling and a flattened group delay or add another 180° to the naturally magnetic cross-coupling producing two rejection notches similar to those shown for a four-element capacitive cross-coupling filter. The cascading of such circular resonators allows a multistage waveguide filter with a number of single cross-coupled quadruplets (similar to meandered combline configuration) to be developed. Figure 12 shows an example of a 6.4 GHz, five-element, circular waveguide cavity filter, having two dual- and one single-mode circular resonators in cascade with a low pass step impedance filter. The near passband response of such a filter is also shown.

Fig. 12 A five-element filter based on three circular waveguide resonators (a) and its response (b).
A practical example of an eight-section, meandered, dielectric resonator filter with two cascaded quadruplets is shown in Figure 13. The use of two quadruplets results in four real frequency transmission zeroes. This filter has a very squared response due to the number of cross-couplings employed and the high Qu factor of the dielectric material giving a sharper passband edge. The inductive couplings between consecutive dielectric resonators are provided by the opening in the partitions, and the capacitive cross-couplings between 1-4 and 5-8 elements are provided by straps/rods suspended inside the dielectric spacers.

Fig. 13 Eight-section meandered dielectric resonator filter with two cascaded quadruplets (a) and its response (b).
A practical example of an eight-section, folded dielectric resonator filter with two cross-couplings, capacitive between third and sixth, and inductive between second and seventh, is shown in Figure 14. Four real frequency transmission zeroes, similar to the six-element filter shown previously, are achieved. The difference in employing cross-couplings in this folded structure versus the aforementioned meandered filter is a slightly flatter group delay response for the folded structure. However, due to the nesting of cross-couplings (that is, one inside the other) and the interdependency of the cross-couplings, the asymmetry of the folded structure is greater and the near band rejection suffers, which makes it harder to tune.

Fig. 14 Eight-section folded dielectric resonator filter with two cross-couplings (a) and its response (b).
An example of a ten-element RX filter duplexed with a six-element TX filter is shown in Figure 15. The TX filter uses a quadruplet to achieve near band rejection goals, while the ten-section RX uses two inductive cascade triplets to achieve the near band rejection goals on the high side. A typical performance of such cross-coupling is also shown.

Fig. 15 Six-element TX/10 element RX channel duplexer (a) and its filter response (b).
Another example of a duplexer, including two six-element filters is shown in Figure 16. Both the TX and RX filters employ a folded configuration and a cross-coupling technique known as the general section. The general section is a well-documented design concept17,18 and can be very useful in achieving the multiple cross-couplings in a minimal package size thus reducing production costs. In this particular example, the six-element folded filters, with the middle four resonators used to employ the general section, illustrate the possibility of simultaneous quadruplet and triplet coupling realized in the four-element section. The general section cross-coupling technique was used here, due to its flexibility in dealing with the stringent rejection requirements and reduction in package size. A typical response plot can also be seen.

Fig. 16 General section-based duplexer (a) and its filter response (b).
Another practical issue is the high power handling capability and ways of eliminating voltage breakdown in high power filtering structures employing cross-couplings. Obviously, the major recommendation to insure voltage breakdown-free operation is maintenance of the safe gaps with the frequency/dimension (FD) product well below critical. Nevertheless, certain steps to minimize the voltage at the capacitive parts implementing cross-couplings (rods, discs, flags, etc.) can be taken when allowed by the electrical response. As was mentioned previously, a triplet capacitive cross-coupling configuration results in a low side transmission notch (zero). Considering a main signal path and assuming a combline filter structure, such as magnetically coupled TEM resonators, the phase gained by the signal at the central frequency between the first and third resonators is approximately 180°, which means that the voltage applied to the capacitive cross-coupling element is approximately twice the voltage at the top of the resonator. Figure 17 is proof of this statement. The cross-coupling shown was used in a TX filter coupling across one resonator (that is, skip 1, triplet). The power gap was insufficient and resulted in arcing from the resonator, which caused the filter failure. There is much to consider when designing cross-couplings into high power filters — material selection for thermal consideration as well as the cross-coupling structure to utilize and also the filter topology to use. To fix the arcing problem, the filter topology and the cross-coupling structure had to be re-designed. The topology was modified to accept a quadruplet in place of a triplet. This reduced the required power gap due to a reduction in voltages between the resonators being cross-coupled (90 x 3° phase). However, the trade-off is a slightly reduced near band rejection.

Fig. 17 The fork type capacitive cross-coupling in a dielectric holder melted by voltage arcing.
An asymmetric cross-coupling, maintaining no significant voltage applied to the capacitive element, is a quintuplet, that is, skip three elements, a capacitive cross-coupling where the propagated signal phase between the cross-coupled resonators is about zero (90 x 4°) (only capacitive cross-couplings are considered because magnetic couplings, loops for instance, naturally sustain no voltage applied). Figure 18 shows the response of two five-element filters, each employing one capacitive cross-coupling, but skipping one and three elements. The minimal signal propagating through the quintuplet cross path is 90° ahead of the main path signal at the center frequency. Again, because of significant phase slope difference between the main and cross paths, the first in-phase/out-of-phase conditions occur inside the passband, below and above the center frequency correspondingly, which did not noticeably affect the response. The second two-signal in-phase/out-of-phase summation is taking place on the skirt below and above the passband, which results in a similar capacitive triplet response, with the exception of degraded skirt selectivity and low side rejection. It should be mentioned that the more elements that are skipped, a smaller capacitive element with greater capacitive gaps is needed to provide the same transmission zero location. The downside of skipping a greater number of elements in general is a weakened skirt selectivity and out-of-band rejection with the same transmission zeroes location caused by a growing imbalance between parts of the signal propagating through the cross (greater) and main paths outside the passband.
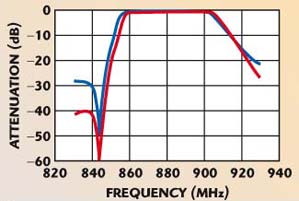
Fig. 18 Two five-element filters with the single capacitive cross-coupling skipping 3 (Pentaplet) and 1 (Triplet) elements.
Useful Software to Make Filter Design and Evaluation Easier
The variety of software available on the market today usually provides an analysis of the given circuit that is a powerful instrument in the hands of knowledgeable engineers, especially in the initial design concept stage. The most known and widely used packages include HP EEsof, Serenade, Microwave Office and Eagleware. All the listed programs offer circuit simulation, optimization and physical filter analysis, based on a closed form solution to specific components for planar topologies. Microwave Office offers an EM simulation for arbitrary planar-type filter topologies. Nevertheless, in the author’s opinion, Eagleware is a better choice when it comes to specific conceptual filter design needs. An important tool offered by Eagleware is a filter synthesis module allowing an instant L-C lumped element traditional filter simulation with the given input parameters, which in its turn becomes a starting point in any filter design task. Following this step, any skilled user can drag, place and connect inductive or capacitive elements between certain L-C resonators in order to achieve the necessary amplitude or group delay responses. Once all the changes are made to the initial circuit, an optimization program can adjust the element values to meet the electrical requirements. Achieving the design task may require a few cycles of cross-coupling element adjustments as well as optimizer runs. In a vast majority of cases, the finalized conceptual electrical design takes no more than one hour to manually synthesize, analyze and display all the required filter parameters (based on an eight-section, two cross-couplings BPF filter). One thing that should be taken into consideration during the simulation procedure is the possibility of physical implementation of the chosen cross-coupling in the final filter realization/ topology (transmission line, resonator types).
Another type of software available on the market, focusing on the aspects of filter design and therefore requiring a good understanding of the filter theory, is a filter simulation/synthesis software such as FILSYN, FILPRO, NTK and the previously mentioned GWT (available only on-line). The authors would like to pay more detailed attention to the package they are familiar with.
The Network Tool Kit Package (NTK) is a rigorous application of a group of filter design techniques collectively known as the insertion loss method. The insertion loss method is mathematically very robust, and has been enhanced by several workers in recent years for microwave filter applications. Although the Insertion Loss Method is general enough for simulation and synthesis of filter networks based on a variety of mathematical functions, the NTK software is based on the implementation of the mathematical functions known as the Generalized Tchebycheff polynomials. Generalized Tchebycheff polynomials allow the specifications of finite frequency transmission zeroes while maintaining the in-band equi-ripple Tchebycheff characteristic.
Network Tool Kit is a two-part software:
1. NTK is a very powerful simulation software allowing the user entire flexibility to vary all major filter parameters in a very user friendly windows screen. In that necessary part of the software, the major input parameters along with frequencies, ripple and Q factor, are finite transmission zeroes and a dispersion model.
2. The NTK extraction program allows the designer to determine exactly the value of every network element (coupling bandwidths between resonators as well as coupling matrix similar to one shown in Table 2) and gives maximum flexibility in the selection of the network configuration.
Figure 19 illustrates an NTK synthesis result of a twelve-element flat group delay filter having identical input data to one shown previously, but exhibiting better symmetry because of the more complex synthesis based on element extraction. The NTK output files contain all necessary output design information such as immittance inverter summary (coupling bandwidths), resonant element values and coupling matrix.

Fig. 19 A 12-element filter synthesized by NTK, its (a) filter response and (b) group delay.
References
- R. Levy, “Filters with Single Transmission Zeros at Real or Imaginary Frequencies,” IEEE Transactions on Microwave Theory and Techniques, Vol. 24, No. 4, April 1973.
- K.T. Jokela, “Narrowband Stripline or Microstrip Filters with Transmission Zeroes at Real and Imaginary Frequencies,” IEEE Transactions on Microwave Theory and Techniques, Vol. 28, No. 6, June 1980.
- G. Preitzenmaier, “Synthesis and Realization of Narrowband Canonical Microwave Bandpass Filters Exhibiting Linear Phase and Transmission Zeros,” IEEE Transactions on Microwave Theory and Techniques, Vol. 30, No. 9, September 1982.
- R. Levy, “Synthesis of General Asymmetric Singly- and Doubly-terminated Cross-coupled Filters,” IEEE Transactions on Microwave Theory and Techniques, Vol. 42, No. 12, December 1994.
- J.H. Cloete, “Tables for Nonminimum-phase Even-degree Low Pass Prototype Networks for the Design of Microwave Linear-phase Filters,” IEEE Transactions on Microwave Theory and Techniques, Vol. 27, No. 2, February 1979.
- A.E. Atia and R.R. Bonetti, “Generalized Dielectric Resonator Filter,” COMSAT Technical Review, Vol. 11, No. 2, Fall 1981.
- R.W. Rhea, “Exploiting Filter Symmetry,” Microwave Journal, Vol. 44, No. 3, March 2001, pp. 100–108.
- R.W. Rhea, “Transmission Zeros in Filter Design,” Applied Microwave and Wireless, January 2001.
- On-line Microwave Filter Design, www.guidedwavetech.com.
- A.E. Williams, “A Four-cavity Elliptic Waveguide Filter,” IEEE Transactions on Microwave Theory and Techniques, Vol. 18, No. 12, December 1970.
- A.E. Atia and A.E. Williams, “New Types of Waveguide Bandpass Filters for Satellite Transponders,” COMSAT Technical Review, Vol. 1, No. 1, Fall 1971.
- A.E. Williams and A.E. Atia, “Dual-mode Canonical Waveguide Filters,” IEEE Microwave Theory and Techniques, Vol. 25, No. 12, December 1977.
- J. Uher, J. Bornemann and U. Rosenberg, Waveguide Components for Antenna Feed Systems: Theory and CAD, Artech House Inc., Norwood, MA, 1993.
- A.E. Atia and A.E. Williams, “Nonminimum-phase Optimum-amplitude Bandpass Waveguide Filters,” IEEE Microwave Theory and Techniques, Vol. 22, No. 4, April 1974.
- A.E. Atia and A.E. Williams, “Narrow Bandpass Waveguide Filters,” IEEE Microwave Theory and Techniques, Vol. 20, No. 4, April 1972.
- R.J. Cameron and J.D. Rhodes, “Asymmetric Realization for Dual-mode Bandpass Filters,” IEEE Microwave Theory and Techniques, Vol. 29, No. 1, January 1981.
- U. Rosenberg and D. Wolk, “Filter Design Using In-line Triple-mode Cavities and Novel Iris Couplings,” IEEE Microwave Theory and Techniques, Vol. 37, No. 12, December 1989.
- T. Reeves, N. van Stight and C. Rossiter, “A Method for the Direct Synthesis of General Section,” IEEE International Microwave Symposium Digest 2001, Vol. 3, pp. 1471–1474.
- R.J. Cameron, “General Prototype Network-synthesis Methods for Microwave Filters,” ESA Journal 1982, Vol. 6.
