This article is the second in a two-part review addressing the history of ultra wideband (UWB) communications and radar. The first part covered UWB communications, and here UWB radars and sensors are addressed. There is overlap in the choice of subcomponents for UWB communications and radar systems, therefore the focus is on the novelties and capabilities offered by UWB radar/sensor systems and specific development requirements. UWB signals offer signal bandwidth delivered in a very short duration of time (high instantaneous bandwidth). UWB radars/sensors also probe the target's optical response more effectively, promise the capability of high resolution target imaging and detection, and, in some application areas, have achieved commercial success in ground, wall and foliage penetration, synthetic aperture radar (SAR) and target imaging. In both reviews, the pioneering, innovative and fundamental contributions to the field are presented.
Ultra wideband or UWB signal is synonymous with impulse, carrier-free, baseband, time domain, nonsinusoidal, orthogonal function and large-relative-bandwidth radio/radar signals. Here, the term UWB is used to include all of these. Contributions to the development of a field addressing UWB RF signals commenced in the late 1960s with the pioneering contributions of Harmuth, Ross, Robbins, van Etten and Russian investigators. In 1974 Morey designed a UWB ground penetrating radar (GPR) system, which was commercialized at Geophysical Survey Systems, Inc. (GSSI). Other subsurface UWB radar designs followed (e.g., Moffat and Puskar, 1976).1 The commercial development of fast sampling receivers (mainly for oscilloscopes) in the late 1960s aided in developing the UWB field. These approaches were imported to UWB designs. In the 1960s both Lawrence Livermore National Laboratory (LLNL) and Los Alamos National Laboratory (LANL) performed original research on pulse transmitters, receivers and antennas.
Thus, by the early 1970s the basic designs for UWB signal systems, for radar or communications, were available and there remained no major impediment to progress in perfecting such systems. After the 1970s, the only innovations in the UWB field could come from improvements in particular instances of subsystems, but not in the overall system concept itself, or even in the overall subsystems' concepts. The basic components were known, including pulse train generators, pulse train modulators, switching pulse train generators, detection receivers and wideband antennas.
Between 1977 to 1989, the USAF had a program in UWB system development headed by Col. J.D. Taylor. At this time, there was already substantial progress in UWB in the former Soviet Union/Russian Federation1 and China,2 which paralleled the progress in the US. There were also very active academic programs (including LLNL, LANL, the University of Michigan, the University of Rochester and Polytechnic University, Brooklyn) which focused on the interesting physics of short pulse transmissions that differed from the physics of continuous or long pulse signals, especially with respect to interactions with matter.4
In 1994, T.E. McEwan, then at LLNL, invented the Micropower Impulse Radar (MIR), which provided for the first time a UWB radar operating at ultra low power, besides being extremely compact and inexpensive (McEwan, 1994, 2000). This was the first UWB radar to operate on only microwatts of battery drain. The methods of reception of this design also permitted for the first time extremely sensitive signal detection.
UWB RADARS/SENSOR FEATURES
Target Response Aspects
A UWB radar or sensor system is perhaps more unconventional than a UWB communications system, not primarily in system components, but in the physics involved in the signal-target interactions. In the UWB radar case, the transmitted signal is shorter in spatial length than the target. Conventionally, the complete radar cross section of any target can be specified by four complex numbers for a given frequency and set of orientation angles in a scattering matrix. In the case of UWB signals, this description of the radar cross section is still valid. However, the number of frequency components in the UWB transmitted signal is large, and different scattering components on the target will not only have different resonant frequencies, but will also, due to their spatial separation on the target, reflect the illuminating short duration UWB signal at different times. Therefore, the UWB signal return is the sum of separate returns spread out discontinuously in time and consists of multiple time-separated scattering matrices, reflecting the separate spatial interactions on the target.
With respect to a conventional radar signal, which, in most cases is longer in spatial extent than the target, the target's scattering matrix is not discontinuous in time. Therefore, with respect to most conventional radar signals, the target is a point scatterer -- in contrast to the case of UWB radar signals, for which the target response decomposes into a collection of individual scattering components.
Using pulse compression techniques and chirped signals, conventional radars can also resolve a target into its individual scattering components, but only if the echo frequency difference Δf between, for example, two individual scattering components, is equal to the reciprocal of the uncompressed pulse width τ or
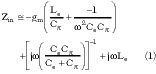
Therefore, if the target subcomponent frequency differences are small, the chirp pulse length τ must be long. If τ is long, then the compression pulsewidth τcomp must be short. This forces the total change frequency over the duration of the uncompressed pulse ΔF to be broad, according to the pulse compression ratio relation
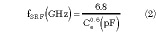
The shorter the compressed pulse, the broader the frequency change:
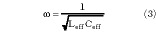
The requirement for a broad frequency sweep dictates a high change in transmitter frequency over the duration of the transmitted pulse τ. The practicality of high rates of change in the transmitter frequency is the delimiter for pulse compression techniques.
Thus, in the case of pulse compression, the frequency difference between target subcomponent echo must be large enough for the echoes to be resolved by a compression filter. UWB radars/sensors are able to resolve those subcomponents without the frequency difference requirement because the returning echoes are already separated in time (nonoverlapping) prior to reception. Therefore, UWB radars/sensors offer an alternative method to high resolution target imaging.
Moreover, depending on the ratio of the spatial/temporal length of the UWB signal to the length of the target, the UWB echo or target response can be one of at least three kinds: early-time (optical) response; resonance response; and late-time response (Cheville and Grischkowsky, 1995, 1997). Whereas the early-time response is target aspect-dependent, resonance and late-time responses are aspect-independent with respect to the harmonic components in the target's response, but aspect-dependent with respect to amplitude of that response. Finally, each scattering component separated-in-time composing the complete UWB response of the target can possess, in varying degree, the three kinds of responses.
The first type of response component, the optical or early-time response, is only present in the case of a fast rise-time signal and only cleanly and commonly seen in the case of UWB radars/sensor signals. The resonance and late-time types of return signal components are also seen in conventional radar. The resonance response of a target is established when the surface currents are present on the target or target components, and the late-time response occurs at the commencement of the decay of the resonance response and after the end of the exciting signal.
The optical or early-time response is more associated with UWB radar. The radar community was first made aware of this target response by Morgan (1984) and Pearson (1984), who pointed out that a forced component, in addition to the damped sinusoidal components (the resonance and the late-time response), is an essential part of the scattered response over the time interval during which an impulsive plane wave is present on the scatterer (see also Van Blaricum, 1991). This means that a transient scattered field cannot be expressed purely as a harmonic response until the scatterer's natural modes are established, that is, until the resonance component is established. The early-time (optical) response of the transient scattered field is characterized by time-varying coefficients determined by local features of the scattering object, and is due to direct physical optic fields, as well as a sum of temporally modulated natural modes. The duration of this early-time response is also equal to the time the wave shape is present on the scatterer. In some instances and orientations, the early-time (optical) response components of the return signal can be of much larger peak amplitude than the resonance and late-time responses.
Radar signals can be characterized not only with respect to duration and spatial extent with respect to the target, but also with respect to the target size L/signal wavelength * ratio. Wavelength- and target-size dependent scattering regimes are as follows: the Rayleigh region, where the signal wavelength is much longer than the size of the target (L/ << 1) and the reflected signal is inversely proportional to the fourth power of the wavelength; the Mie or resonance region, in which the wavelength of the signal is of the same order of magnitude as the size of the target (0.5 < L/
<< 1) and the reflected signal is inversely proportional to the fourth power of the wavelength; the Mie or resonance region, in which the wavelength of the signal is of the same order of magnitude as the size of the target (0.5 < L/ < 10); and the optical scattering regions, in which the wavelength of the signal is much shorter than the target (L/
< 10); and the optical scattering regions, in which the wavelength of the signal is much shorter than the target (L/ >10), and, in the case of some target shapes, the target reflectivity can be frequency independent. UWB addresses the scattering regimes of the resonance and optical regions, and the three return signal components discussed above fall in those regimes.
>10), and, in the case of some target shapes, the target reflectivity can be frequency independent. UWB addresses the scattering regimes of the resonance and optical regions, and the three return signal components discussed above fall in those regimes.
Radars and Sensors
There are marked differences between a conventional and a so-called UWB radar/sensor, that is, an ultrashort pulse radar/sensor, with respect to signal design, emitters, receivers and forms of processing. There are also design differences between a UWB radar and a UWB sensor. In fact, the designer of a UWB radar must decide up-front whether to design a UWB radar or sensor, both of which are different from conventional radars. Whereas the objective of a UWB radar is to provide target detection, the objective of a UWB sensor is to provide information concerning the target, that is, target identification. A UWB radar has more relaxed receiver requirements than a UWB sensor.
Despite the unfortunate UWB labeling, the fundamental distinguishing feature of the UWB emitted signal is not that it is ultra wideband, but that it is ultrashort in time and spatially shorter than the length of most targets. The ultra wideband nature of a UWB signal is, in fact, a derivative from its ultrashortness. The fact that the UWB signal is shorter than the length of a target means, as previously explained, that the target's response decomposes into its individual scattering components and those returning signal components are spread out discontinuously in time. In the case of conventional radar, the signal is usually longer spatially than the length of the target, which means the target response is not spread out discontinuously in time. In the case of a target irradiated by a UWB signal, the target becomes a collection or aggregate of point scatterers, all of which are associated with subcomponents of the whole target. Thus the term, UWB radar pulse (or ultrashort RF pulse) can only be adequately defined relative to a stated target spatial extent L. Considering a pulse which is ultrashort, and therefore UWB, with respect to a Boeing 747 may not be so with respect to a cruise missile, or even with respect to a subcomponent such as an engine on a Boeing 747. Presently there is no agreed absolute duration, bandwidth, or Q defining a UWB signal. In this article, and in the definition of UWB technology, the applicable advice (Taylor, 1995, p. 3) is "Because ultra wideband is a new term, it is best to look for the writer's definition or to determine the meaning in context and the accompanying details, and then apply the mathematical descriptions loosely."
Some helpful definitions are
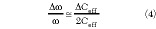
However, not all definitions of UWB radar based on these definitions are useful. An OSD/DARPA (1990) panel provided a UWB signal definition using fractional bandwidth: "Ultra wideband radar is any radar whose fractional bandwidth is greater than 0.25, regardless of the center frequency or the signal time-bandwidth product." However, in discarding any reference to the duration of the pulse from the definition, the OSD/DARPA panel essentially over-generalized the label, permitting it to apply to any signal which occupied a fractional bandwidth greater than 0.25, regardless of the time over which that occupancy occurs. Thus a chirped signal, the instantaneous bandwidth of which is narrow, but which occupies over one second or more a fractional bandwidth greater than 0.25, would be considered UWB, according to the panel's definition. Selection of a 0.25 fractional bandwidth boundary is, of course, arbitrary; the panel's definition neither captures the ultrashort nature of the "UWB" signal or the ultrawide instantaneous bandwidth resulting from that ultrashortness. Nevertheless, the label "UWB" has been attached to this technology, and it is perhaps not too late to remove it. (The laser physics community was not so plagued by inappropriate labels. Ultrashort, and hence ultrawideband and light pulses, are called ultrashort pulses -- cf. Hopkins and Sibbett (2000).)
If one conceives of the target reflection of an emitted signal and the return of the transformed signal to the emitter in terms of a signal input to a system and signal output from a system, then the reflectance properties of the target are described by a transfer function (in the frequency domain) or impulse response (in the time domain). In these system theory terms, a target's transfer function in the case of conventional radar is usually, at steady state, a unitary response function of a duration associated with target size; however, in the case of UWB radar it is a time series of aggregate responses associated with the size, orientation and material composition of the target subcomponents. In fact, the UWB emitted signal, target reflectance (transfer) and signal reception can be considered an informational channel, in which intelligence about the target is impressed on the emitted signal by the target at the commencement of that channel, and that intelligence or information arrives at the receiving antenna, or the end of the channel.
Given this intelligence about the target arriving at the receiving antenna, the design engineer has the aforementioned choice: either to trade that received intelligence into a high signal-to-noise (S/N) detecting radar, or preserve that intelligence (baseband signal) in a sensor capable of target identification, characterization and imaging.
UWB RECEIVERS
Because of the UWB signal's fast rise-time, the UWB receiver must have appropriate bandwidth. The receiver must have a sampling rate adequate to preserve the peak power against averaging or integration. Detection of the signal then proceeds by either threshold or correlation methods.
Taylor and Kisenwether (1995) contrast the narrowband receiver design bandwidth requirement based on the pulse duration * as
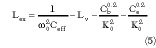
for which, in the case of conventional pulse detection, the detector section must operate up to frequency

while UWB detector must operate up to frequency
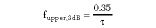
where tr is the pulse rise-time. In fact, the UWB receiver detection circuit is driven by the pulse's rise-time and not the pulse width. Note that the time derivative of the receiving antenna forcing function
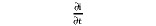
where i is the receiver current in amperes, is the E-field strength (volts/meter) of the received signal before reception, such that
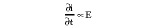
The increased bandwidth associated with the received signal rise-time increases the noise in the receiver, and because UWB signals have a wide relative bandwidth, signal components at the low and high bounds are processed with different fidelity, dispersion and loss.
Taylor and Kisenwether (1995) point out that, whereas in designing conventional receivers it is conventional to describe the signal in units of volts (joules/I * s), or watts (joules/s), in the case of UWB receivers the signal is conceived in terms of volts * second (joules/I), or watts * sec2 (joules * s). These signal definitions reveal that there is a clear requirement for the UWB receiver to measure the signal energy, and not the average signal energy. In other words, there is a requirement to measure the signal peak energy or power, not the average signal energy or power.
As stated previously, the UWB radar system designer can choose between designing a receiver for a target-detecting UWB radar or a target-discriminating and imaging UWB sensor. If the intention is to design a UWB sensor, then the waveform of the UWB return signal must be preserved; however, in the case of a UWB radar, it need not be preserved. In the case of a UWB sensor, a homodyne receiver is appropriate, and in the case of a UWB radar, either a homodyne or a superheterodyne receiver is appropriate (cf. Barrett, 1995b, Taylor and Kisenwether, 1995).
Detection can be performed by threshold detection or correlation detection (with a requirement of a SNR at least 10 dB). In the detection stage of a UWB radar, nonlinear threshold detection is adequate (cf. Robbins, 1972), but in the detection stage of a UWB sensor, the requirement to preserve the original return signal waveform requires sufficient bandwidth associated with expected signal rise-/fall-times, that is, preservation of the modulations impressed on the signal by the target.
Taylor and Kisenwhether (1995) showed that for threshold detection: in the case of the conventional narrowband receiver, the performance degrades (minimum detectable signal (units: watts or volts) is greater) as the receiver bandwidth increases because of increase broadband noise.
In the case of the UWB receiver, the performance improves (minimum detectable signal (units: watts * sec2 or volts * sec) is less) as the receiver bandwidth increases because more signal transient energy is captured, and because of the assumption that the signal bandwidth is greater than the noise bandwidth. (If the signal bandwidth is not greater than the noise bandwidth, then this argument would need amending.)
Therefore, whereas the design objective for conventional, narrowband receivers is to decrease the bandwidth to improve narrowband signal sensitivity by rejecting impulse signals, the design objective for the UWB receiver is to maximize the bandwidth to improve impulse signal sensitivity, and thereby neglect frequency selectivity.
If there is no requirement to preserve the waveform of the UWB return signal, that is, the case of a UWB radar, not sensor, then correlation techniques can be used for detection. There are two forms of correlation detection of the individual pulse auto-correlation and cross-correlation. Auto-correlation correlates the received UWB signal with a preserved copy of the transmitted signal. Due to the fact that the target and path impresses modulations on the transmitted signal and increases its duration, in the case of auto-correlation there would need to be some modification of the preserved signal according to predictions of those modifications. In the case of cross-correlation a reference waveform is decided in advance of transmission. In both cases the appropriate statistics to apply in the case of UWB signals is that of a random, nonstationary processes.
There is extensive literature on the processing of pulse trains. It is well-known that the power spectral density of a pulse train is proportional to the absolute value of the Fourier transform of the single pulse (despite claims by some UWB proponents that changing the interpulse intervals in a pulse train, or dithering, flattens all of the power density spectrum). In addition, the phase information in the Fourier transform of the individual pulse is not retained in the auto-correlation, therefore the power density spectrum of the pulse train does not have a unique solution (that is, the same power spectral density can be obtained from different collections of signals).
Neglecting these drawbacks and having obtained the power spectral density (PSD) of a pulse train, it can be used in various ways. For example, if the pulse train is impressed with Barker or complementary (Golay) codes, then the instantaneous power of each pulse can be reduced to achieve the same total energy interaction with the target (over the train), assuming the target is not performing maneuvers over a time scale comparable to that of the pulse train and the target's response is not extremely aspect-dependent. Also, time and spatial resolution can be improved by pulse compression over the length of the pulse train using well-known methods. In addition, the SNR of the return signal can be improved by the same pulse compression methods.
It should be noted that, given the intention to design a fast UWB receiver preserving the modulations impressed by the target on the signal, the heavy continuous processing burden requiring 20 GHz sampling rates must be addressed. These sampling rates force the designer to consider receiver designs based digital phosphor and SiGe technology, for example.
GROUND, WALL AND FOLIAGE PENETRATING RADARS
UWB radars for ground and wall penetration offer the obvious advantage of not requiring simultaneously transmitting while receiving from close proximity targets. Almost all GPR systems presently operate at or below 1 GHz primarily because the attenuation of the signal by the ground increases with frequency (see Daniels et al., 1988). However, two other mechanisms may also be operating: the ground can also be a dispersive medium, and therefore the low(er) frequencies in the return signal at the surface can either be due to low pass filtering or medium dispersion; and the relaxation times of various ground media are comparatively long (Barrett, 1991, 1995b), which means short pulse envelope effects can occur. The relative influence of these different mechanisms is unknown at the present time.
The presence of water in the ground which can influence ground conductivity is important because, according to Maxwell's theory, dielectric constant and conductivity always occur in combination, and thus together will affect the propagation of waves in matter (cf. King and Smith, 1981). The phase shift in a propagating wave is related to both the medium's effective permittivity and effective conductivity (Ulriksen, 1982). Furthermore, the relaxation time of water in soils is displaced to a lower frequency than that in bulk water (Hoekstra and Delaney, 1979), and there appears to be two closely spaced relaxation times, rather than one (De Loor, 1983).
In GPRs, a number of techniques are followed to remove noise and artifacts (Schneider, 1978; Safar, 1985; Osumi and Ueno, 1988; Yeung and Evans, 1988), a major artifact being due to the footprint size of the beam projected from a moving vehicle being cone shaped and wide so that there are multiple interactions with a single stationary below-the-surface target, hence multiple overlapping spatial returns.
The UWB signal, which delivers a wide bandwidth in a short time, is compatible with the requirements for a SAR (LaHaie, 1992). Aircraft are equipped with UWB SARs, and the UWB signals penetrate foliage (FOPEN) to reveal objects concealed in forest and vegetation (Vickers, 1992), utilizing the capability of UWB pulses to deliver a wide bandwidth in a short period of time.
RUSSIAN SYSTEMS
There has been extensive development of UWB systems and subsystems in the former Soviet Union and the present Russian Federation. This work arose out of programs to improve power systems in the 1950s. Even at this stage, the difference was noted between conventional continuous wave signal description methods and ultrashort pulse methods (e.g., Zernov, 1951). The simplicity of the methods of time domain analysis for short pulse UWB signals, as opposed to continuous, steady state signals, was described by Kharcevitch (1952).
Initially, radio pulses of nanosecond duration were generated using traveling wave tube modulation (Astanin and Kardo-Sysoev, 2000). In 1957 Astanin, while at the A. Mozjaisky Military Air Force Academy, developed an X-band 0.5 ns duration transmitter for waveguide study. A receiver-correlator with a T-bridge waveguide and mechanically controlled delay was used (Astanin, 1964). At the same time, at the Radioelectronics Institute of the USSR Academy of Science, Kobzarev and collaborators conducted tests on indoor ranges of ultrashort pulse high resolution radars. These constituted the first stage of development of UWB systems in Russia (Astanin and Kardo-Sysoev, 2000).
The next stage of development utilized fast semiconductor switches, beginning with Shatz (1963) and continuing at the Ioffe Physico-Technical Institute. As in the United States, progress was facilitated by the availability of fast sampling oscilloscopes -- initially at Novgorod (Rjabinin) and at Vilnius, Lithuania (Efimtchik and Levitas). The theoretical basis for time-scale transformation procedures was developed by Naidenov (1978) and eventually 10 GHz sampling oscilloscopes were developed. At this time, the applications were in GPR (Finkelstein, 1994, Shirman, 1991). It was realized that UWB signals constitute a separate class of signals and that Doppler, in the case of ultrashort pulse trains, becomes not a measure of phase, but a time scale transformation (Astanin and Dorsky, 1988; Astanin and Kostelev, 1989). These investigations resulted in the formulation of a time domain analysis of signals, or Radar Target Characteristics involving target impulse response, ramp response, as well as signal shape and signal structure description (Astanin et al., 1994).
While the different nature of ultrashort pulse or UWB systems from conventional radars became enveloped in rancor and controversy in the US, it was first realized in Russia that ultrashort (shorter than target length) pulses deliver more target parameter information, such as target state and orientation, than do conventional long (longer than target length) pulse systems. This realization led to the addressing of target classification and imaging, as well as to the theoretical issues of ill-posed problem solution (Tikhonov and Arsenin, 1986; Kostelev, 1984). The theory of ill-posed problems became a new branch of mathematics and its basic results were first obtained by Soviet mathematicians. Treatments of ultrashort pulse signal returns also used tomographic methods (Kononov, 1992) and wavelets (Astanin and Kostylev, 1997). The development of ultrashort pulse transmitters even included picosecond and femtosecond pulse transmitters (Glebovitch et al., 1984). In recent years, there have been major theoretical advances (for example, Feld (1991), Borisov (1995) and Kostylev (2000)), as well as advances in mine detection (Astanin et al., 2000).
From a subcomponent standpoint, the obvious solutions were used, as they were in the US. For example, Astanin and Kostylev (1989) summarized the earlier work addressing a time domain transmitter and receiver and observed that "Generators based on avalanche transistors are widely used." Meleshko (1987), in a section titled "Time Correlation of Pulsed Signals," states "In devices which implement the first, simplest method, correlation is performed at the moment when the leading edge of the input pulse crosses the constant threshold." Furthermore, many circuit designs of Tracking-Threshold Shapers are shown, indicating that the use of signal edge detection was commonplace.
Varganov et al. (1985, p. 5) provided one definition of superwideband signals, namely Δf/f = 1, and described a number of methods for transmitting and receiving impulse signals. Presently, there is a variety of definitions of UWB. According to OSD/DARPA (1990), UWB devices must have a 20 dB fractional bandwidth of at least 0.25, where the fractional bandwidth is 2(fH fL )/(fH +fL ), where fH is the upper frequency of the 20 dB emission point and fL is the lower frequency of the 20 dB emission point. The center frequency is defined as (fH + fL )/2.
The Federal Communications Commission has provisionally adopted the OSD/DARPA (1990) definition, but with a modification: UWB devices are defined as any devices where the fractional bandwidth is greater than 0.25 "or occupies 1.5 GHz or more of spectrum." The FCC also provisionally proposes to base the UWB definition on the 10 dB bandwidth, rather than the 20 dB bandwidth. Furthermore, the FCC provisionally adopts the OSD/DARPA (1990) definition of center frequency.
In the late 1960s and early 1970s Kardo-Sysoev discovered a new method for switching modular thyristors called avalanche injection (AI). Even at these early dates, switching times of ~10 ns at 1 kV and > 100 A were achieved (Kardo-Sysoev et al., 1976). The group at the Ioffe Physico-Technical Institute, St. Petersburg, and headed by Kardo-Sysoev, also pioneered the development of delayed ionization switches called SAS or silicon avalanche shapers (Kardo-Sysoev et al., 1981). The SAS required high rise-time triggering (>1012 V/s) and have been used as shaping heads for thyratrons and vacuum tubes. A second generation of devices -- drift step recovery diodes (DSRD) -- were capable of supplying that triggering (Kardo-Sysoev et al., 1985). These developments made possible solid state pulsers with greater than megawatt peak powers and 0.1 ns fronts. The devices were used in the late 1980s in the development of an anti-stealth UWB pulsed phased antenna array -- a development which was ultimately cancelled on the collapse of the USSR (Kardo-Sysoev, 2000).
Presently, the Ioffe Physico-Technical Institute group offers an extremely powerful pulser combining all the earlier work in the form of compressor cells. The compressor cells are modulator thyristors for 100 ns cells, DSRDs for nanosecond cells and SASs for subnanosecond cells. Russian ground penetrating radars include those of I.M. Finkelshtein (Riga) and V.E. Kotenkov (Moscow).
HIGHER-ORDER SIGNAL PROCESSING
The radar cross-section of a target has a complex relationship to the physical size of a target and depends on the target dimensions, shape and material composition, as well as the illumination angle of the target and the frequency and polarization of the incident waveform. Unlike human visual perception of images, which is based on noncoherent optics, radar is based on coherent optics. Because of the coherent nature of the target return reflectances, there is no way to distinguish the true target scattering responses present at the target and an interference pattern of target responses at a distance, that is, at the receiving antenna. Therefore, in the case of target identification, radar is always dealing with a coherent optics interference pattern, not an incoherent optics image. One may choose to call that interference pattern an image of the target, but it is not an image in the visual, incoherent optics sense. Despite the difference we shall not distinguish here between coherent and noncoherent imaging.
The problem of recognizing a target from all aspect angles has received much attention (cf Noel, 1991; Taylor, 1995; Harmuth, 1995) has presented methods using sequences of character codes and pulse train summing over time delay, resulting in preservation of transmitter signatures, regardless of target aspect. Next, two more approaches to the target aspect-dependence problem will be examined.
SINGULARITY EXPANSION METHOD
The singularity expansion method (SEM) estimates the target scattering matrix in Laplace transform form. As is well-known, the Laplace transform of the target's impulse response, that is, its transfer function, can, in many cases, be represented or summarized in terms of the poles and sinks of the function. This pole and sink summary can provide a signature permitting target identification. The presupposition of the approach is that the target's transfer function is linear -- which it generally is, but not always. (Note, the white noise analysis approach does not assume that the target's transfer function is linear. In fact, this approach is a method for detecting nonlinearities.) The great advantage of Laplace analysis is that the transient, as well as the harmonic, nature of the target's transfer function can be characterized. The history of the development of the SEM approach is covered in Van Blaricum (1995).
The SEM method is further able to characterize the target response in terms of the optical, natural resonance and standing waves. The poles resonances of a target are aspect-independent, but the ability to couple to them is not. If the SEM application is applied predominately to the natural resonances across aspect angles, the result can still be encouraging, but the method requires close attention to using a processing method that uses the return signal components from target resonances excitable at all angles, and neglects those resonances excitable only at certain angles.
WHITE NOISE ANALYSIS
The Wiener functional expansion is a general method for analyzing nonlinear systems that have a finite memory. A system is nonlinear if the principle of superposition does not apply to the system, either because the system's frequency aggregate dispersion response is not a linear additive function of the individual single frequency dispersion responses (dispersive nonlinearity) or because the system's aggregate amplitude response is not a linear additive function of the individual single pulse amplitude responses (intensity-dependent nonlinearity).
In the case of pulsed excitations, a system's nonlinearity can be described as an influence of the past input to a system on the response of the system to a present input to the system, which attenuates with time, that is, the system has memory. For example, the late-time response of a target exhibits a form of memory of the driving signal no longer present on the target. The decay time or offset time of a receiver is also an indication of this form of memory. Thus, there is an inter-pulse temporal threshold, that is, the driving pulses must be separated less than a certain inter-pulse interval Δt for a memory influence to occur. However, after that threshold is crossed (Δt < threshold), the response of the system adds. The addition can be instantaneously linear, that is, the principle of superposition can apply to the instantaneous addition, but due to the aforementioned temporal threshold, and the response decay over time, the system is nonlinear according to the previous definition, that is, the principle of superposition does not apply over time. There is no system response addition when the inter-pulse interval Δt is greater than the threshold and the amount of addition of the first response to the second response is a function of the system's decay time. This kind of system response is sometimes called temporal summation.
With those definitions in mind, methods due to Volterra and Wiener, which underpin white noise analysis, will be explored. Volterra (1959) showed that the transfer function or impulse response of a nonlinear, time invariant, finite memory, analytic system can generally be characterized by a generalization of the Taylor series of multiple arguments known as the Volterra series. Next, Wiener (1942, 1958; Lee, 1964) showed that if the input to an unknown system is Gaussian white noise and if the Volterra series components are mutually orthogonal, it is possible to calculate the Volterra description of a system in terms of a series of kernel functions, now known as the Wiener kernels. Wiener constructed a hierarchy of these functionals (or functions of functions) that are orthogonal to each other with white noise as the stimulus to the system under consideration. The Wiener series representation of a system's transfer function/impulse response is related to the Volterra series; however, as it is an orthogonal series, it has a stronger convergence than the Volterra series. Once the set of Wiener kernels are estimated, a system's transfer function/impulse response is completely characterized. The Wiener kernels were difficult to compute until Lee and Shetzen (1965) showed how these kernels can be evaluated by well understood cross-correlation techniques.
White noise analysis methods are general and describe the transfer function/impulse response of any unknown system (cf. Marmarelis and Marmarelis, 1978; Barrett, 1978). More recently, these methods have also been applied to characterize the transfer function/impulse response of targets to RF signals.
An approach derived from white noise analysis is bispectral analysis (Marmarelis and Sheby, 1988; Jouny and Walton, 1991), which involves triple auto-correlations and can detect return signals with skewed amplitude probability distributions. Another derived approach is that of (Wiener) kernel analysis (Marmarelis et al., 1995) involving the characterization of the target transfer function/impulse response in terms of higher-order functionals (convolutional integrals) or Wiener kernels. The higher-order kernels, higher than the first, describe the system nonlinearities, and the full set of kernels constitutes a generalized impulse response function. The transmitted signals are based on pseudorandom, or continuous-time broadband random processes with zero mean (Marmarelis et al., 1995). The technique permits noise suppression, provides phase information and also signal reconstruction. Preliminary results characterizing materials and targets using these techniques have been promising.
TIME-FREQUENCY APPROACHES TO HIGH RESOLUTION TARGET IDENTIFICATION
A strict adherence to merely time or frequency methods alone removes from consideration powerful methods for characterizing and identifying targets. These new methods fall in the general category of time-frequency methods. If a signal is decomposed into a time-frequency wavelet or wavelet packet representation, and multivariable correlations (for example, across time or frequency) are performed on that two-dimensional representation, then signal response differences between targets are made explicit and can be quantified by correlation.
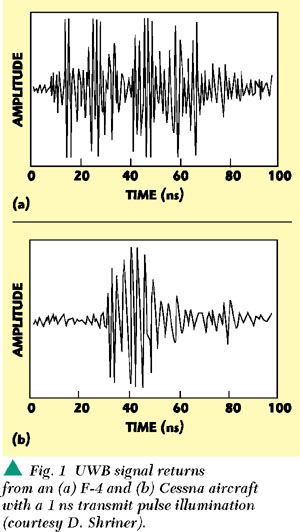
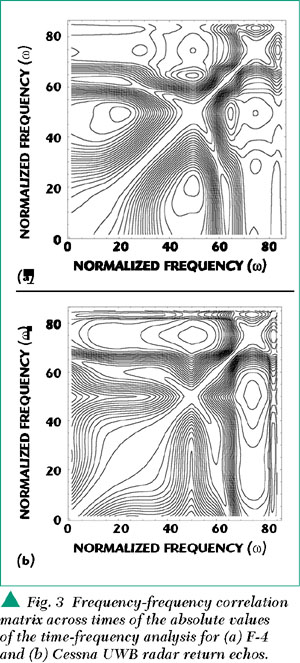
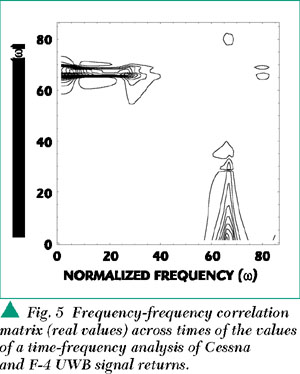
To illustrate, two representative UWB target return signals are shown in Figure 1. Figure 2 shows these signals in a time-frequency representational form. Figure 3 shows frequency-frequency correlations of the time-frequency matrices. (Values across the diagonal are identical. Values along the diagonal are unity.) Figure 4 shows correlations of the time content of the frequency bins and covariances. The correlations of set frequency components of the two return signals over time are low and provide a characterization of differences between the two targets' instantaneous frequency response over time. These data provide indication of time-and-frequency differences in the signal return from the two targets. Each target return signal is shown to have a distinctive signature or fingerprint not apparent in the raw signal. In the case of these particular UWB return signals, the frequencies at set times are correlated negatively at 0.025, but the times of set frequencies are correlated positively at 0.67. After this processing the difference between the two signal returns can be easily distinguished, as shown in Figure 5, and the two UWB target signal returns, which at first appeared very similar, show a small correlation in most instances over the analyzed time-frequency plane. Thus, the time-frequency decomposition processing provides a powerful method for making explicit discriminating differences between target signal returns, and permits target recognition and identification on the basis of pattern.
|
|
|
|
|
|
|
|
|
TIME-FREQUENCY APPROACHES TO HIGH RESOLUTION TARGET DETECTION
The high resolution target detection capabilities of UWB radars/sensors have advantages and a related disadvantage. The disadvantage is that a transmitted UWB pulse will interact with a variety of targets. Therefore, signal returns from targets of no interest will crowd the receiver as undesired interfering noise, or clutter, lowering the designated target's SNR. Of course, what is designated clutter, and what is designated a target, are matters of choice. Furthermore, the choice can change. Nonetheless, given a designated target, clutter lowers the SNR of the return signal from the chosen or designated target.
A method for enhancing the designated target SNR in the presence of designated clutter, which also permits changes in the choice of designated target and clutter, was suggested by Barrett (1996) -- the matched pulse or matched adaptive time-frequency packet (MAP) signal approach. The MAP method is a time-frequency frequency approach and requires a priori information about the impulse response of the designated target, or classes of target.
Figure 6 shows the general MAP procedure. Suppose a requirement exists to enhance the SNR selectively for two targets, or classes of targets: target 1 and target 2. Further, suppose that targets 1 and 2 will also serve to provide the clutter signal return for each other, that is, the choice of which is designated target and clutter can be changed. In this scenario, the impulse response of both targets must be obtained (A and B) using a UWB signal; for example, a monocycle, as the transmit pulse. The received signal in each case is treated as the impulse response of each target. These received signals are then time reversed to form their complex conjugate, and for purposes of exposition, called matched pulse 1 and matched pulse 2. Information concerning a target's impulse response and thereby of its matched pulse constitutes a priori information about a target or class of targets.

In a second operational scenario (C and D), the matched pulse for target 1 is transmitted and the return signal from targets 1 and 2 received. Neglecting issues of the scattering matrix's polarization and aspect dependence, according to systems theory the return signal from target 1 is enhanced (C), and depending on the relative orthogonality of target 2 impulse response with respect to that of target 1, the return signal from target 2 is diminished (D). That is, the matched pulse 1 enhances the SNR of the signal return from target 1.
Conversely, (E and F), the matched pulse for target 2 is transmitted and the return signal from targets 1 and 2 received. Again, neglecting issues of the scattering matrix's polarization and aspect dependence, according to systems theory the return signal from target 2 is enhanced (E), and depending on the relative orthogonality of target 1 impulse response with respect to that of target 2, the return signal from target 1 is diminished (F). That is, the matched pulse 2 enhances the SNR of the signal return from target 2.
This method can be utilized to reduce clutter to discriminate otherwise very similar targets or classes of targets on the basis of small differences between the targets' scattering matrices, and to provide early warning of known threatening targets or classes of targets.
SUMMARY OBSERVATIONS
A number of summary observations can be made concerning this historical account of the development of UWB radars/sensors. UWB radar systems have been in the commercial world since the 1970s. They have been successfully used in ground-, wall- and foliage-penetration, position-location, collision warning for avoidance, fluid level detection, intruder detection and vehicle radar measurements. UWB radar is employed in SAR for FOPEN and ground mapping. Future planned applications include distance and air-bag proximity measurements and backup warning, road and runway inspection, breathing and heart monitoring, RF identification and camera auto-focus.
Conventionally, target identification is achieved by using a broadband signal to excite a target's resonant frequencies, or for precise range resolution resolving all the major scatterers on the target. A swept frequency waveform can conceivably excite all of a target's resonances; however, those excitations are over a period of time which would preclude precise range resolution. A UWB radar promises the achievement of both aims in a single system and a single signal waveform.
Pulse power sources were discussed in the first review. To achieve target detection/identification at substantial range, a UWB radar/sensor requires to transmit sufficient energy on target. A number of methods designed to illuminate the target with sufficient energy at substantial range have been proposed (cf. Noel, 1991; Taylor, 1995). The proposed methods include arrays of emitters and trains of pulses in conjunction with correlation receivers.
The antennas used or proposed for efficient transmission in UWB radar/sensor systems include loaded dipoles, TEM horns, biconicals, ridged horns, spiral and the large current antenna. All have merits and disadvantages.
The field of UWB radar/sensing, although still having a contentious history, has been less plagued -- so far -- with exaggerated performance claims in the public press, and invalid priority and originality claims, than has UWB communications. The virtues of UWB radar/sensing are demonstrable and apparent, while the virtues of UWB communications remain obscure at this time.
ACKNOWLEDGMENT
This work was originally presented at Progress In Electromagnetics Symposium 2000 (PIERS2000), Cambridge, MA, July 2000. *
References
1. A partial list of the many companies offering GPRs or GPR services includes Sensors and Software Inc., Geonics Ltd., Pulse Radar Inc., GeoRadar Inc., Penetradar Corp., ENSCO Inc. and Era Aviation.
2. cf. Chernousov, 1965a,b, 1969; Glebovich et al., 1984; Varganov et al., 1985; Meleshko, 1987; Astanin and Kostylev, 1989, 1992, 1997; Astanin et al., 1994; Stryukov et al., 1989; Zernov, 1991a,b; Sodin, 1991, 1992; Immoreev, 1991, 1997, 1998; Immoreev and Zivlin, 1992; Immoreev and Teliatnikov, 1997; Immoreev and Fedotov, 1998; Osipov, 1995; Krymscy et al., 1995; Bunkin et al., 1995; Efanov et al., 1997; Kardo-Sysoev, 1997.
3. cf. Harmuth (1981), pp. 388-9.
4. cf. Miller, 1986; Barrett, 1991; Barrett, 1995a, Bertoni et al., 1993; Carin and Felsen, 1995; Baum et al., 1997; Heyman and Mandelbaum, 1999.
Click here for a comprehensive reference list for both Part I and Part II of this article.